Abstract

Thermal Conductivity and diffusivity were measured using two associated dynamic methods; the transient plane source (TPS), and the dynamic plane source (DPS). One of the advantages with these techniques is the possibility to extract all thermos-physical parameters, the thermal conductivity, thermal diffusivity and specific heat from one single transient recording. These two methods use a probe that is technically a “resistive element”, as the heat source and temperature sensor. A description of the main features and the principles on which these methods are based will be emphasized. The thermal conductivity and diffusivity of composite polymer materials such as polystyrene and polymethlmethacrylate are reported. With emphasis on the thermal properties of such materials, a variety of implementation and classification regarding heat conduction will be discussed. A note on the difficulties of measurements of composite materials involve nanoparticle suspensions will be highlighted.

Keywords: Thermal conductivity; Thermal diffusivity; Composites; Polymers; Dynamic Methods; Nanoparticle suspensions.

Introduction

The thermal properties of composite polymeric materials are of practical importance because they govern the temperature-rise magnitude, the anisotropy [1-2] of heat flow and, the thermo-mechanical reliability in the thermal designs for modern manufacturing of such materials.

In addition, computer simulation of polymer flow dynamics [3-4] during the filling and post-filling stages in manufacturing require the knowledge of thermal properties. Therefore, more sophisticated models need to be developed to explain fully the relationship between molecular structure and anisotropic behavior for various kinds of polymers [4b].

In this work, we will deal with all thermo physical properties that are directly related to heat conduction such as thermal conductivity λ, thermal diffusivity κ, and specific heat Cp. Behavior of thermophysical properties of any kind of materials can be correlated to their mechanical and structural changes. The relatively small change in structure can be correlated with reasonable change in the value of the specific heat. The other two parameters, thermal conductivity and thermal diffusivity, are transport parameters related to the ability of material to transport heat. In general, the thermal properties of amorphous polymers depends on many factors, such as chemical constituents, structure, type and strength of defects or structure faults, strength of bonding, molecular density distribution, molecular weight of side groups, processing conditions and temperature. Depending on temperature, different polymers undergo different structural changes leading to changes in their phases. According to Jäckel and Scheibner [5], the low temperature thermal behavior is strongly influenced by the anisotropy nature of the bonding forces; the phonon vibration spectrum is governed by three-dimensional interchain oscillations at low temperature and the molecular motions inside the polymer chains dominate at elevated temperatures. Data about such materials at the different thermodynamic stages (phases), within different temperature ranges, usually are not available; therefore, a direct measurement of thermal properties of these materials is highly demanded for the use in specific applications.

The reliability of a specific method to measure thermophysical properties is given by several factors, such as the speed of operation, the required accuracy and performance under various environmental conditions, the physical nature of material, and the geometry of the available sample. However, in most methods the main concern is to obtain a controlled heat flow in a prescribed direction, such that the actual boundary conditions in the experiment agree with those assumed in the theory.

The transient dynamic methods are a class of methods for measurements of thermal properties of materials. The principle of these methods is simple. The sample is initially kept at thermal equilibrium, and then a small disturbance is applied on the sample in a form of a short heating pulse. The change in temperature is monitored at one or more points during the time of measurement. The thermal diffusivity is then evaluated by correlating the experimental temperature measurements with the theoretical relationship obtained from the solution of the differential heat equation. Carslaw and Jaeger (1959) [6] give solutions for different experimental arrangements at various initial and boundary conditions.

The most simple transient methods, the hot-wire or the hot-strip each uses a line heat source (wire or strip) that is embedded in the specimen initially kept at uniform temperature. With this methods, it is possible to measure both the heat input and the temperature changes, and then the thermal conductivity or both (only in hot strip case) conductivity and diffusivity are simultaneously determined.

The transient plane-source (TPS) method is originally based on hot strip method and characterized by the transient temperature rise of a probe “resistive element” at constant energy input. The probe is a plane double spiral, Figure 1. Used as both constant heat source and temperature sensor. The probe (TPS-sensor) is made of a Ni strip 10 µm thick covered from both sides with thin insulating layer made of Kapton, different sizes of sensors were used in this work. The duration of the current pulse (time of measurement) is usually in the order of few seconds. Measurement is simply performed by recording the voltage (resistance/temperature) variations across the sensor that is supplied with pulsed electrical current [7-8].

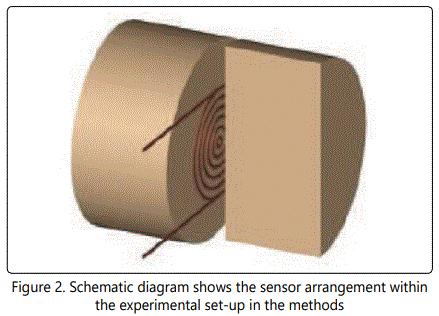
In the dynamic plane source (DPS) method [9-10] we have used the TPS-sensor as a plane source placed inside a medium in such a way so that its experimental arrangement resembles a one-dimensional heat flow, as shown in Figure 2.

In this work, we are using these methods to measure the thermal conductivity, diffusivity, and specific heat of composite polymeric materials.

Experiment

TPS method

The experiment is simply performed by recording the voltage variations over the TPS-sensor while its temperature is slightly raised by a constant electrical current pulse. The sensor is clamped between two identical cylinders or square-shaped pieces to insure a good thermal contact between the sensor and the sample pieces, see Figure 1. The duration of the current pulse (time of measurements) are within several seconds to few minutes. The short measuring time is an advantage that can significantly reduce the risk of thermal degradation, which is a common characteristic of polymer materials. Furthermore, during this short period, care is taken not to increase the temperature in the samples more than few degrees, since a small temperature increase (small gradient) will reduce errors that may mask some phase transition which occur within narrow temperature regions during measurements.

The theory of the method is based on a three-dimensional heat flow inside the sample, which can be regarded as an infinite medium, if the time of the transient recording is ended before the thermal wave reaches the boundaries of the sample. The time dependent resistance of the TPS-sensor during the transient recording can be expressed as

where R₀ (≈ 4 Ωat room temperature) is the resistance of the TPS element before the transient recording has been initiated, α is the temperature coefficient of resistance (TCR), for the TPS sensor (α≈ 4.0 x 10^-3 K^-1 at room temperature) and ∆T(t) is the time dependent temperature increase of the TPS sensor. Depending on the temperature range of interest, the TCR values for the TPS-sensor are determined within that particular temperature-range from separate calibration procedures by means of different thermometers such as a Pt-thermometer.

The assessment of ∆T(t) in the heater depends on the power output in the TPS sensor, the design parameters of the sensor and the thermal transport properties of the surrounding sample. For the disk-shaped sensor ∆T(t) is given by the following equation, from which the thermal conductivity and diffusivity can be obtained

where, P₀ is the total output power, l is the thermal conductivity of the sample, and a is the radius of the sensor. D(τ) is the theoretical expression of the time dependent temperature increase, which describes the conducting pattern of the disk shaped sensor, assuming that the disk consists of a number m of concentric ring sources [7-8], see Figure 1. For convenience the mean temperature change of the sensor is defined in terms of the non-dimensional variable τ, where 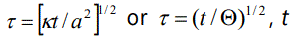is the time measured from the start of the transient heating, τ =a² / κ is the characteristic time, andκ is the thermal diffusivity of the sample. The specific heat is related to these parameters through the density ρ via the relation ρ C_p = λ/κ

DPS method

The main features distinguishing DPS from the TPS can be summarized as:

(i) DPS is arranged for a one-dimensional heat flow into a finite sample, which is in a contact with very good heat conducting material (heat sink) such as copper [10].

(ii) DPS also works in the time region where the sample is treated as a finite medium and is not restricted only to the time region where the sample is regarded as infinite medium.

(iii) DPS has the potential to give λ, κ, and ρC_p from a single measurement even if the experimental arrangement resembles a one-dimensional heat flow.

The temperature increase in the plane x = 0, (0< x <l<), as a function of time will be given by

Where q is the total output power per unit area dissipated by the heater, 2l is the length of the sample (see Figure 2) and Θ = l²ρ is the characteristic time of the sample. F(Θ,t) is a theoretical expression of the time dependent temperature increase [9], whose value depends on the measuring time and the sample characteristics as follows:

For times 0 < t < 0.3Θ , F(Θ,t) = (t/Θ)^1/2 from which the value of effusivity ξ=λ/√κ is obtained, then for times t ≥ 2Θ, ∆T(t) will approach the value ql/2λ and λ can be readily obtained.

For times t ≥ 0.5Θ, the natural logarithm of the derivative of ∆T(t) can be expressed [10]. as:

Then ρC_p can be obtained from the intercept of the graph between ln[d∆(t)/dt] and t.

Finally, a further check for the obtained value of ρC_p can be verified via the data consistency relation ρC_p = λ/κ.

Results and Discussion

The TPS and DPS methods has been tested on four samples (shown in Table 1) with thermal conductivity ranging from 0.028 to 0.403 W/m K, The choice of these samples was because its thermal properties are within the range of typical polymeric materials. The values shown in the table are the means of five independent measurements for the TPS technique and means of six independent measurements for the DPS technique. These data are not more than 5% from the literature data [11-14] Even though, these data where taken at ambient temperatures the methods have the potential to probe thermal properties during temperature variations and phase transition [15-16].

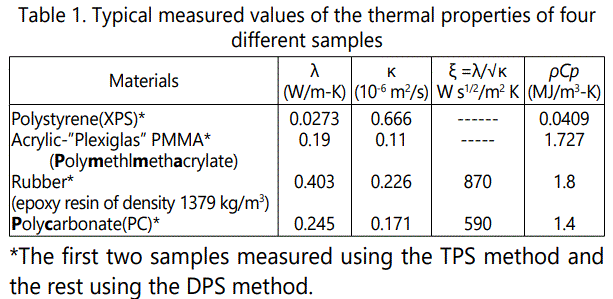
The data reliability was tested by using these two methods. Data were measured on commercial PERSPEX of 30 and 50 mm in diameter. For the TPS method we have used three probes of different sizes (Figure 1). Data were evaluated using standard evaluation analysis within the conclusions of the theory of sensitivity coefficients for given parameters and differences [17].

The final difference of the averaged values for thermal conductivity and specific heat are less than 2% and for thermal diffusivity is less than 4%.These data are in reasonable coincidence comparing to scattering of data that were collected from literature and were put to the data consistency relation λ=κρc. Most of the literature data are obtained using steady state methods that are giving just one parameter. The scatter of the data from literature is usually bigger than 10% for each parameter [18-19].

The effect of temperature change on thermal properties of composite polymeric materials is complex and it depends on several factors such as the type of polymer, degree of polymerization, the structure of the matrix etc. For example, in non-conducting polymers as temperature increases, the chain movements could have two opposite folds: one that is related to the size and number of micro-voids created by these movements and the other is related to increasing the chain alignment and arrangement within the matrix. The former reduces the thermal conduction due to defects and the later enhances the conduction due to alignment and stacking of chains. Thus with this two fold effect thermally non-conducting polymers may show different conduction behavior and can be classified into two main groups. Polymers with heavier chain segments and long branches will resist movements and as a result reduces thermal conduction and polymers with lighter chain segments and short branches that have high degree of polymerization which will enhance thermal conduction.

In addition, these types of polymers can be mixed with fillers to make polymer composites, then predicting the thermal behavior of their polymer composite will never be an easy task. In such composite material, thermal properties often vary from batch to batch (or from time to time), it could also vary under various environmental conditions, or during recycling processes. Therefore, developing a technique to measure thermal properties under various thermodynamic conditions within relatively short measuring time would significantly reduce the risk of thermal degradation and other rate dependence effects that tend to create significant spread in the measured values of thermal conductivity and diffusivity.

Both currently discussed methods have been developed to meet such demands. These methods have the potential to be utilized in research and development mapping applications where structural changes may be empirically correlated with the measured values of thermal conductivity, particularly, in cases where structural stability, structural control and/or the presence of defects are related to the thermal transport properties. These methods are non-destructive and relatively fast techniques that can be used as quality control assurance devices along the production line in manufacturing of components, especially, when information in production manufacturing processes with higher quality requirements is needed. In material research and developing, these methods can be used, when the knowledge of material behavior is required even during the steps of manufacturing.

Furthermore, composite polymeric materials including composites with inorganic fillers, the value of thermal diffusivity could reach the value of the same order as some metals [20-23] and it is significantly related to the molecular and the super molecular structures in binary composites and polymer blends.a recent review article by Cahill et al. [24] discusses the effect of interfaces in composite materials that involve nanoparticle suspensions. In this way, the interfacial thermal transport may involve a complicated process in which the phonons carrying most of the heat across the interface are not necessary the phonons carrying the heat in the bulk or along the fiber, which in turn makes phonon-phonon coupling within each material forming the interface an important factor. This will require more advances in experimental techniques to provide improved control and characterization of interface structure to explore the thermal

Conductivity of Composites filled with nano-fillers which is quite different and not well understood yet [25].

Finally, composite polymers are promising materials for improving the physical and mechanical properties, which cannot be achieved by single polymers.

Therefore, as described above, thermal properties are sensitively affected by change in the microstructure of materials and these measurements can be considered as probes to explore the change in microstructure of the blends, which do not require special physical or chemical modifications of the sample specimens.

Conclusions

The discussed Dynamic Transient Methods (TPS, DPS) are very sensitive techniques for measurements of thermos-physical properties of solids within a wide temperature range and they have the potential to probe these properties during structure relaxation and phase transformations. The main advantage is that the dynamic method can give all three thermos-physical parameters, comparing to steady state methods.

These methods are non-destructive techniques because due to the following reasons:

(i) During the experiment the temperature of the sample is practically not changed (∆T < 1 K), Thus, resulted parameters λ, κ and c_p could be used as a measure for the quality control even during manufacturing processes, as well as the stability in time.

(ii) The same sample can be used for next type of physical measurements (for example, electrical, or optical) which can be correlated to the thermal properties to deduce meaningful comparison and or to develop a theoretical formalism correlating the structure and physical properties.

The thermos-physical parameters thermal conductivity, diffusivity and specific heat of polymeric materials are important parameters regarding safety issues for human (production against fire) or environmental issues during degradation of polymeric materials due temperature variations.

References

1. Piraux L, Kinany-Alaoui M, Issi JP. Thermal conductivity and thermopower enhancement in magnetic acceptor graphite intercalation compounds. Solid State Comm. 1989; 70: 427. doi: 10.1016/0038-1098(88)90823-X   
2. Benerus DC. Phys Rev Lett. 1999; 82:366.   
3. Hieber CA, Chiang HH. Technical Manual No. TM-5 Supplement, Cornell Injection Moldin Program. 1986.   
4. Hieber CA, Injection & Compression Molding Fundamentals. A. I. Isayev (ed), Marcel Dekker, New York. 1987.
   
   
   4b. Kurabayashi K. Anisotropic Thermal Properties of Solid Polymers. International Journal of Thermo physics. 2001; 22: 1. doi: 10.1023/A:1006728223978   
5. Jäckel M, Scheibner W. Some Peculiarities in Thermal and Relaxation Properties of Polymers and Related Materials at Low Temperatures. Phys Sta Sol. 1992; 134: 391. doi: 10.1002/pssa.2211340209   
6. Carslow HS, Jaeger JC. Conduction of heat in solids. 2nd Ed. Oxford University Press. 1959.   
7. Suleiman BM. Physics Department, Chalmers University of Technology, Gothenburg, Sweden. 1994.   
8. Gustafsson SE. Transient plane source techniques for thermal conductivity and thermal diffusivity measurements of solid materials. Rev Sci Instrum. 1991; 62: 797. doi: 10.1063/1.1142087   
9. Karawacki E, Suleiman BM. Meas Sci Technol. 1991; 2: 744   
10. Karawacki E, Suleiman BM, Izhar ul-Haq, Bui-Thi Nhi. An extension to the dynamic plane source technique for measuring thermal conductivity, thermal diffusivity, and specific heat of dielectric solids. Rev. Sci. Instrum. 1992; 63; 4390. doi: 10.1063/1.1143739   
11. Log T, Gustafsson SE. Transient plane source (TPS) technique for measuring thermal transport properties of building materials. J Fire and Materials. 1995; 19: 34. doi: 10.1002/fam.810190107   
12. Graves RS, Wysocki DC. Insulation Materials Testing and Applications, ASTM STP 1116, (Eds), Philadelphia. 1991.   
13. Drysdale D. An Introduction to Fire Dynamics. John Wiley, New York. 1990.   
14. Waszink JH, Hannes GE. Heat Transfer Conf. 9th Intern. Jerusalem. 1990.   
15. Suleiman BM, Börjesson L, Berastegui P. Effect of cobalt doping on thermal conductivity of YBa2Cu3O7−δsuperconductor. Phys. Rev. 1996; B53(9): 5901. doi: 10.1103/PhysRevB.53.5901   
16. Suleiman BM, Karawacki E, Gustafsson SE. J. Mater. Res. 1994; 9: 1895.   
17. Kubicar L, Bohac V. Proceeding of 25th International Thermal Conductivity Conference/12th International Thermal Expansion Symposium, Pittsburgh, USA. 1997.   
18. Bohac V, Gustavsson MK, Kubicar L, Gustafsson SE. Parameter estimations for measurements of thermal transport properties with the hot disk thermal constants analyzer. Rev Sci Instrum. 2000; 712452.   
19. Kubicar L, Bohac V. Proceedings of the 1st International Conference on Thermophysical Properties of Materials, Peter Hing(Ed), Singapore. 1999.   
20. Takeo Araki, Qui Tran-Cong. Structure and properties of composite polymeric Materials. Mitsuhiro Shibayama(Eds). 1998; 293.   
21. Gun-Ho Kim, Dongwook Lee, Apoorv Shanker, Lei Shao, Min Sang Kwon, et al. High thermal conductivity in amorphous polymer blends by engineered interchain interactions. Nature Materials. 2015; 14: 295-300. doi: 10.1038/nmat4141   
22. Teng Zhang, Tengfei Luo. Role of Chain Morphology and Stiffness in Thermal Conductivity of Amorphous Polymers. J Phys Chem B. 2016; 120(4): 803-812. doi: 10.1021/acs.jpcb.5b09955   
23. Vadivelu MA, Ramesh Kumar C, Girish Joshi M. Polymer composites for thermal management: a review. Journal of Composite Interfaces. 2016; 23(9): 847-872. doi: 10.1080/09276440.2016.1176853   
24. Cahill DG, Braun Paul V, Chen G, Clarke DR, Fan S, et al. Nanoscale thermal transport II. Applied Physics Reviews. 2014; 1: 011305.   
25. Congliang Huang, Xin Qian, Ronggui Yang, Cornell University library, https://arxiv.org/abs/1805.05561 . Materials Science (cond-mat.mtrl-sci), Cite as: arXiv: 1805.05561. cond-mat.mtrl-sci v1. 2018.