Abstract

EM theory started from electricity and its current, as the carriers or objects, mediated by the fields and potentials. In the opposite sense, the fields are formal features of the potentials, limited by the carriers. Apart from the central Coulomb’s law, similar Ampere’s law is here generalized. The radial – static and transverse – kinetic, are thus supplemented by longitudinal – dynamic forces. The fields are introduced in the three ways: as the evident forces, via the object densities and by analogy of the potentials with fluid mechanics. As the simplest basic set, the two algebraic relations of J. J. Thomson operate by the two moving fields. Instead of the parallel or hierarchical processes, they form a causal loop with the constitutive field relations. The spatial derivatives of the algebraic pair give the four differential forms, wider from Maxwell’s equations. The elimination of excessive, and explanation of remaining terms, convincingly relate the two sets. Maxwell’s equations are finally presented in Einstein’s tensor form, concerning 4D space.

Keywords: Field, Potential, Static, Kinetic, Dynamic

Introduction

By analogy with gravitation, EM theory formerly started by Coulomb’s law for static interaction of two electric charges. The similar Ampere’s law, for magnetic interaction of two moving charges, as the elementary currents, has not been formulated in general. Its special case is restricted to the transverse plane containing the two charges, and moving with them. Even as such, it is successfully applied to the line conductors and their fields. Its application to the oblique position of a moving dipole implies the torque acting on this dipole. Not only that such torque has never been practically confirmed, but is theoretically doubtful. Without the general Ampere’s law – merely here completed, EM theory demanded some other formal approaches.

Fluid mechanics generalized Newtonian laws from discrete to distributed quantities. Instead of such inductive development, EM field theory is founded by analogy, on the basis of Maxwell’s set, intuitively derived from the technical practice. As the new mathematics and its abstract application were not habituated by the contemporaries, this Maxwell’s challenge was difficult to understand and accept. From his set Maxwell derived the known wave equation, thus predicting EM waves. Hertz’ empirical verification of these waves, with some arrangement of the equations, was sufficient for the wide acceptance of Maxwell’s theory, without insight into the essence.

In the meantime, J. J. Thomson proposed the considerably simpler and logically clearer pair of algebraic relations, treating the field motions – instead of their variations. Despite the smaller number of the simpler equations, there was difficult to follow the motions of invisible fields, unlike their variations accessible by the resting instruments, at the typical points at least. Not elaborated in practice, Thomson’s relations have been suppressed by Hertz’ affirmation of Maxwell’s theory. Only L. Landau presented them, but without any discussion. The other expositions of EM theory do not even this. On the other hand, these relations are the basis of the transformations of the fields and coordinates, obeying the formal invariance of Maxwell’s set.

At least some of differential equations have their algebraic equivalences. The particular inductive procedures consist of the original hits, without a general approach strictly elaborated. For 150 years of Maxwell’s equations, there is unknown any attempt of their algebraic resolution. The special technical situations are successfully treated by computers. However, the application of the two differential operations to Thomson’s pair gives the four differential forms, wider from Maxwell’s set. By elimination of the excessive, with explanation of other terms, this job is here finalized. The formal procedures further illuminate the serious physical relations. The indirect inductive approach to this matter is already presented in the references.

EM Fields

The application of the relativity principle and convective field derivatives enable the consistent foundation of EM theory. These two concepts can be commonly presented:

Here v is the object speed, and V – that of the field A. The vector field gradient, as the tensor, multiplied by the vector also gives the vector. The object motion causes relative, but that of the field gives the opposite, convective field variations, each of them proportional with the field gradient. The spatial motions and temporal variations are thus related. EM theory has been founded on electricity (q), as the abstract bipolar substance. Its volume density gives the scalar field, Q = ∂q/∂v, forming respective current: J = VQ. Its divergence gives the continuity equation (2). Owing to the inert particles, their free motion is uniform, without acceleration or the speed divergence. The former term thus annuls, and latter one, as the convective field derivative, gives the temporal variations

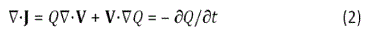
Faraday had introducing the force fields empirically, by the torques (3) and force differences (4) affecting respective dipoles. Though not immediately formulated, these equations adequately express the direct empirical impressions. Electric or magnetic dipoles, directed by the torques (3) along the fields, present the abstract field lines, as the force directions. Thus oriented dipoles are drawn into the stronger fields (4).

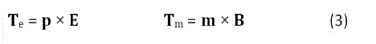
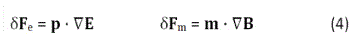
The symmetry of these equations gives the impression of the parallel electric and magnetic phenomena. However, unlike an electric dipole (p = ql), as the polar vector determined by two opposite poles separated by a distance (l), the magnetic dipole or moment (m) cannot be anyhow resolved. Though predicted by analogy, the free magnetic poles have never been separated, but the division of one, ever gives the two shorter dipoles. However, the rotation of an electric dipole around one its pole gives the magnetic moment, as the axial vector, m = p × v, announcing the hierarchy of EM phenomena. The circular electric currents carry toroidal magnetic fields, or vice versa.

The field of an electric dipole starts from its positive, and terminates on the negative poles. The medium disturbance, as the global field integral, is opposite to the dipole: d = – p. The electric dipoles thus orient against external fields, and these fields are usually weaker at material media. However, the magnetic field of a current contour is opposite inside and outside this contour. The magnetic disturbance, h = m, is added to the external field, thus increasing it at matter. This is the evident asymmetry of the two EM phenomena.

By volume densities of the dipoles or medium disturbances, Maxwell introduced the rational fields, e.g. polarization (P) and displacement of electricity (D). Such fields are related by (5), and these with the force fields – by (6).

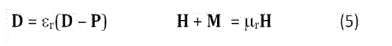
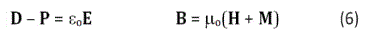
The relative electric and magnetic factors (_r ) relate the total and vacuum field components, but respective vacuum factors (_o) dimensionally reconcile the two field types being independently introduced. The dimensionless relative factors equal to units at the space without matter. The equivalent vacuum medium, of the unknown essence and structure, had been also understood. Its negation in modern physics fails in any interpretation of EM fields and respective waves. The newer similar notions, as the quantum or Higgs’ field, are also equally abstract.

The vertical substitutions of the field difference and sum give the two constitutive relations (7), hiding the field asymmetry. In each of them, respective total field is proportional with its vacuum component, via the total constants. However, the former relation expresses the rational over respective force fields, just oppositely to the latter of them.

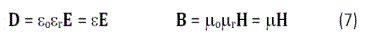
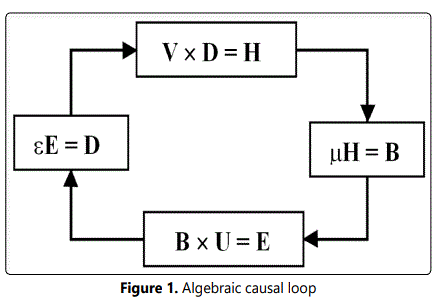
Instead of the symmetry or hierarchy, these equations point to a causal loop, as the field relations. Missing the middle phase of this consideration, consisting of (5) & (6), these two equations further enhance the impression of symmetry, announced by (3) & (4). The two EM constants in the basic sets, instead of a field pair, further hide the essential asymmetry.

Algebraic Relations

Instead of Maxwell’s differential equations, we here start with Thomson’s algebraic relations (8), as the simplest basic set. Here V is the speed of electric, and U – of magnetic fields. At least for their distinction, the former relation we call kinetic, but latter dynamic one. Namely, latter of them modifies the motion expressed by the former. In both of them, the motion of one total, represents or produces the other vacuum fields. The former relation operates by the rational, but latter by force fields. The opposite orders of the fields and speeds, in the cross-products, express their essential asymmetry.

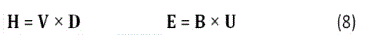
The kinetic relation links the vector densities of the two medium disturbances. However, applied to a rotating electric, it would give the infinite magnetic fields, as the unacceptable result. For this reason, it has been ignored.

The dynamic relation describes respective induction, noticed empirically. A magnet moving with its field causes the electric induction in the field domain. It is also applicable to the rotating magnetic field, as the sum of the resting periodical components. In practice, it is usually added (9) to the equivalent kinetic field (10), affecting moving electricity:

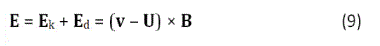
Here v is the object speed, and U – that of the field. The principle of relativity is thus understood, concerning the mutual motion of the object and field. In this manner, the dynamic Thomson’s relation has been also hidden.

The algebraic pairs (7) & (8) form the causal loop (Fig 1). The two asymmetries supplement each other. EM theory thus appears as a closed scientific system, operating by the two field pairs, irrespective of any material carriers.

According to Maxwell’s & Einstein’s relations (11), Pointing’s cross-products of the vacuum or total fields relate the moving densities of the energy and mass (12).

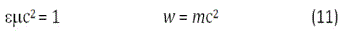
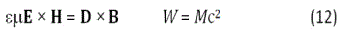
At a free space without explicit matter, the two relative factors equal to units, and the propagation reaches the vacuum wave speed: c_o ² = 1/e_om_o. With respect to the relative factors – in principle greater from units, the effective speed through matter is usually smaller. In some special cases, of so called dispersive media, this ratio may be inverse one.

In comparison with sound and other known waves, the two constants look alike structural elasticity (e) and mass density (m). With respect to the vacuum factors, some medium – enabling EM waves, is unavoidable. Though the explicit matter predominates inside massive bodies, this medium is omnipresent throughout the cosmic space, thus possibly explaining the predominance of the implicit matter, in cosmos as a whole.

Central Forces

The application of (8a) to the central electric field (13a) of a moving electric charge (q) gives respective magnetic field (13b). Avoiding the problematic kinetic relation, this result is obtained by the integration along a line conductor. The kinetic force (10), affecting the electricity moving through magnetic field, gives the special case of Ampere’s law (14).

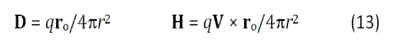
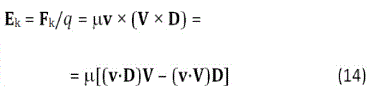
This force is here formally resolved into its axial and radial components. Unlike the former component stretching the particle, the latter of them tends to the radial compression. However, their ellipsoidal sum gives the unacceptable torque on a moving electric dipole, not practically confirmed. The opposite axial forces mutually cancel along the line conductors, and this equation then gives the acceptable results.

The reference of the object speed may be irrelevant: owing to the magnetic field carried by respective conductor, the relative and absolute speeds are equal. However, in the general case (15), the absolute speed need be substituted by relative one (v – U), thus also applying the dynamic relation (8b).

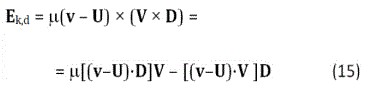
However, the magnetic field motion around a punctual charge is problematic. Its rest or motion with the particle would cancel the magnetic force or produce the cumulative sequence of EM processes (8), both equally unacceptable. There remains a possibility of its transverse motion (16a). The zero axial force is satisfied by (16b), where the conditional equality concerns the common motion of the carrier and object.

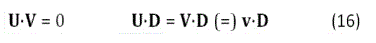
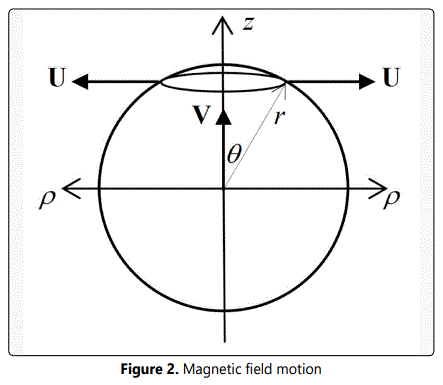
The regular equality is expressible in the scalar forms (17), where θ is the polar angle. The transverse convective derivative applied to the central potential (38a) – on Fig 2, gives the same result. With respect to the magnetic field independent of its own objects, this result is general. At least effectively, the circular field lines expand in the front, and shrink behind the particle, with the instantaneous rest in the equatorial plane. In this position, the result (14) is also valid. The conditions (16) substituted into (15) finally give the general Ampere’s law (18).

The two sine terms present the kinetic forces (14). The third term expresses the axial dynamic induction, due to the transverse field expansion or shrink. In fact, the medium is compressed and accelerated – in the front, and vice versa behind the particle. The induction law (40) applied to the convective derivative of (38b) just expresses this. At common motion of the two charges the first term annuls, reducing (18) to (19).

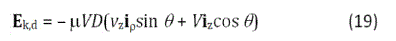
The kinetic forces do not affect resting electricity. Therefore, the dynamic induction subtracted from the central static field (20a) gives the ellipsoidal result on a resting instrument, irrespective of the even pressure upon the moving carrier. At the common motion, with sin2 θ + cos2 θ = 1, the central force (20b) excludes the torque on a moving dipole.

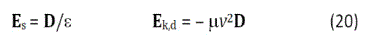
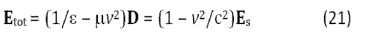
At least some of differential equations have their algebraic The total force (21), affecting a moving charge, thus consists of the static, kinetic and dynamic components. This central sum tends to naught approaching the speed c, just while the mass of the particle is growing into infinity.

Differential Equations

The two algebraic relations operate by the two EM fields, but differential equations – by their spatial and temporal derivatives. Therefore, the differential analysis of the former is the way for its relation with latter sets. In this sense, the routine application of the operation divergence to the algebraic pair gives the two following differential forms:

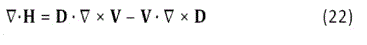
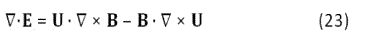
Without the speed derivatives, these forms are comparable with respective Maxwell’s equations (24) & (25), thus restricted to the two homogeneous speeds, at the stationary EM processes. This formal condition just points to the field inertia, so far being ascribed to their material carriers.

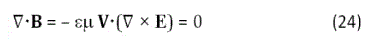
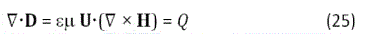
The middle expressions represent the scalar fields. The axial motion of a circular field vortex forms the sources of the other EM field. In the absence of such electric vortex (24) turns into the trivial equation, expressing the closed field lines and excluding the existence of free magnetic poles.

The electric field terminals, in the static equation (25),have been understood as the charge density: Q = ∂q/∂v. Alike a black hole affecting surrounding stars, a charge particle is expected in the center of the radial electric field. Therefore, its reality is founded on the spatial location, unlike magnetic poles which cannot be even located in space. A central static field may be understood as the dynamic induction (8b) at the axial motion of circular magnetic vortices along t-axis.

On the other hand, the operation curl applied to the algebraic pair (8) gives (26) & (27). The terms with the speed derivatives are here already missed. The middle field divergences may be substituted by the two above equations. The two remaining terms, as the convective field derivatives, give the opposite – temporal field variations. The magnetic vortices, in the kinetic equation (26), embrace electric currents, as the motion of free and/or bound electricity. Similar electric vortex, in the dynamic equation (27), acts along the variable current, against possible acceleration of the moving electricity.

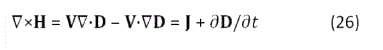
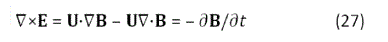
Thus completed Maxwell’s set, missing the speed derivatives, is restricted to the stationary processes. With respect to the magnetic – produced by moving electric fields (8a), the acceleration of electricity is taken into account.

The diagram (Fig. 3) presents the differential causal relations. The initial asymmetry of Thomson’s pair is here increased by the different realities of the electric and magnetic charges.

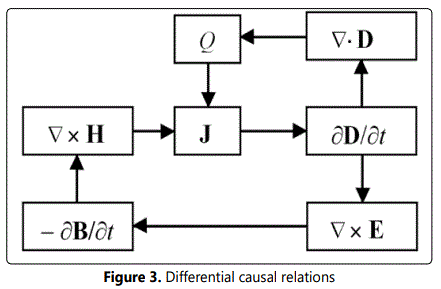
The separation of the static from dynamic electric fields is presented by the feedback on the main causal loop. It expresses the formal production of electricity, as the apparent field carriers. Starting from the electricity assumed in advance, EM theory has been treated as the open scientific system.

Tensor Forms

Einstein condensed Maxwell’s set into the two tensor forms, describing the 4D relations. Unlike his indirect procedure – via EM potentials, the direct transfer is more transparent at least. In this aim, Maxwell’s set is a little accommodated (28)÷(31). The free electricity – moving from the past into future – represents the fourth current component (28). The temporal field derivatives, as the gradients along the metrical t-axis, are replaced to the left of (29) & (31). Each of these vector equations is resolvable into the scalar triples. The two subsets, of the four equations each, are expressible by the two tensor forms (32).

The left form operates by the rational, as the products of the charge density with kinematical quantities, but right one – by the force fields, possibly introduced from potentials (35b) & (37a). The tensor terms are thus determined:

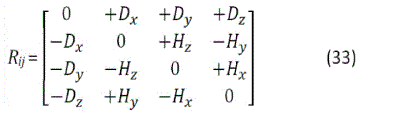
The index i = (t, x, y, z) here denotes the ordinal numbers of the equations in the subsets, and the sums concern the index j ≠ i. The two field pairs seem to be inversely related with variation of a spatial distance. Therefore, one of them has been treated as covariant, and other – as contra-variant vectors. However, owing to the medium disturbances usually proportional with respective forces, this apparent distinction is excessive.

With the metrical sense of temporal axis, in (29) & (31), the natural units (ε_o = 1 = μ_o ) are here understood. Einstein used, at his time actual, units of Heaviside (ε_o = 1/c = μ_o ), with the factor1/c in the two first columns. Their opposite signs, following from the initial asymmetry of (8), substituted by imaginary unit, as the average, ascribe the imaginary sense to t-axis

EM Potentials

In the fluidic model, the static force opposite to the energy gradient equals to the fluid pressure, as the energy density (35a). Respective field, as the specific force, is equally related with the potential, as the specific energy (35b).

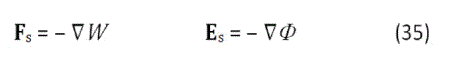
Carried by electric current (J = VQ), the moving static just forms respective kinetic potentials (36a). Unlike the electricity and its current, being directly related, the two potentials are mediated by EM constants. Apart from the static pressure (Φ), the disturbance depends on the medium elasticity (e), as well as the moving mass – on its own density (m). With respect to its equivalence with (8a), the relation (36a) has been also ignored. The equivalent continuity equation (36b), proposed by L. Lorentz, is still accepted at least conditionally.

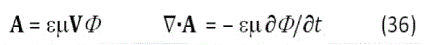
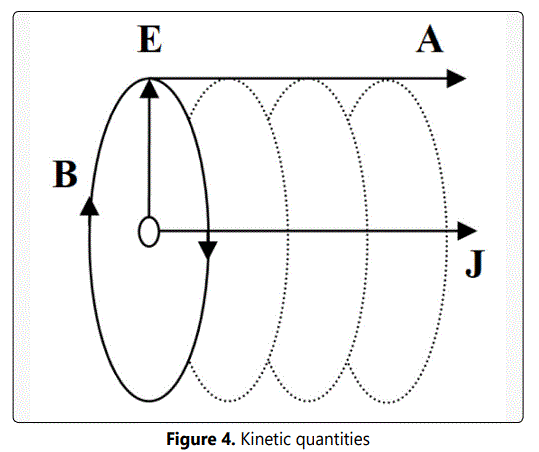
Unlike the elementary magnetic field (13b) related to a moving charge, it is generally defined by the kinetic potential (37a), implying the trivial equation (30).The transverse kinetic force affecting moving electricity (37b) reminds of the curve path of a spinning ball at the fluidic flow.

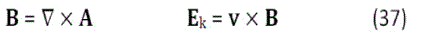
Figure 4 presents mutual relations of the static and kinetic quantities. The equipotent static spheres, cut by the transverse planes (Fig. 2), trace the magnetic field lines, as the isohypses (37a) of the kinetic potential (36a). Closed into themselves, they exclude the free magnetic poles, on the terminals.

Inversely to (35b) & (37a), the convenient integrations of the two central fields (13) give respective two potentials (38). These two particular cases also obey the general mutual relations (36). Unlike the two central fields (13), the first radius degree – in these nominators, point to the energies cylindrically distributed around the temporal or a spatial axis.

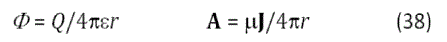
According to Einstein’s and Maxwell’s relations (11), the force action law (39) just implies respective dynamic induction (40). Kinetic potential is proportional with linear momentum density. Its temporal derivative may be treated as the dynamic potential. And finally, curl applied to (40) gives the dynamic equation (27), as the equivalent consequence.

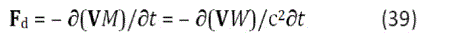
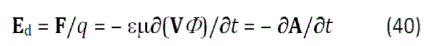
The three EM interactions – static (35b), kinetic (37b) and dynamic (40) ones – thus essentially represent respective fluid-mechanical forces, at the vacuum medium.

Discussion

Ctg-function (17) gives the infinite field speed, approaching the zero polar angle. According to the modern views at least, there is no greater speeds than that of light.

As the ratio of the two transverse projections on the sphere, this function effectively grows from the naught up to infinity. Alike the rotational sum of resting components, this apparent motion also implies the dynamic effect.

Apart from relativity principle, Einstein’s equation is also here applied. On the other hand, the vector variance, from the same theory of relativity, is renounced.

EM theory preceded the relativity. These two theories form a causal loop, alike the two EM fields. Therefore, they legitimately supplement and/or correct each other.

By gradual affirmation of the vacuum medium, the material basis of physics is here called in question, including the particles, as the carriers of the material features.

Classical physics relies on mass and electricity, as the mere concepts, ascribed to material particles. However, the ascriptions do not explain the concept essences.

Resolving certain forgotten problems, the new ones are here opened, undermining the established views.

Only the clear problems can be resolved. The reexamination confirms good, but changes bad views.

Despite the numerous expositions of EM theory, only your own references are here mentioned.

This article substitutes the standard expositions. Even if these latter noticed some problems, they did not resolve them, thus not deserving the role of references.

Conclusions

Starting by the algebraic relations, as the simplest basic set, the three remaining sets are completed: central laws, differential equations and tensor forms. Thus mutually equivalent, these four sets supplement each other in application.

The relativity principle applied to the mutual (object-field) motion generalizes Ampere’s law to a moving dipole, irrespective of its angular position. The restriction to the transverse plane or to line conductors is thus transcended.

The wider sense of the algebraic from differential sets is here resolved by the reduction to the uniform field motion, in the both sets. This is argued by the field inertia, otherwise being ascribed to the assumed material carriers.

A particle is manifest and interacts by its field. There is no any proof of existence of a hard particle body, distinct from this field. In the final instance, a particle is the center at least, something as a knot of the surrounding field.

The force transfer is here also exceeded. Instead of the direct action at a distance, or the successive transfer through space, the algebraic relations describe the local field actions, at each spatial point and temporal instant separately.

Starting by electricity of a vague essence, EM theory seemed to be an open scientific system. The algebraic and differential causal loops close this system. The static feedback is formally producing the apparent electricity.

References

1. Mišković B. Neoclassical Physics. LAP LAMBERT Academic Publishing. 2019.   
2. Mišković B. Essential Overview of EM Theory. Springer. 2013.   
3. Mišković B. Inductive Elaboration of EM Theory.   
4. Mišković B. Deductive Exposition of EM Theory.   
5. Miskovic BV. Curvilinear Cosmology. Int J Cosmol Astron Astrophys. 2023; 5(2): 231-236. doi: 10.18689/ijcaa-1000143