Categorization of Metalloboranes using Skeletal Numbers

doi:10.18689/ijcr-1000105

Research Article

Kironde Road, Kyadondo Block 224, Plot 5387, Kampala, Uganda

*Corresponding author: Enos Masheija Rwantale Kiremire, Professor, Kironde Road, Kyadondo Block 224, Plot 5387, Kampala, Uganda, E-mail: kiremire15@yahoo.com

Received: February 20, 2019 Accepted: February 25, 2019 Published: March 1, 2019

Citation: Kiremire EM. Categorization of Metalloboranes using Skeletal Numbers. Int J Chem Res. 2018; 1(1): 24-34. doi: 10.18689/ijcr-1000105

Copyright: © 2019 The Author(s). This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Download PDF

Abstract

Metalloboranes have been analyzed and categorized using skeletal numbers. Skeletal numbers are extremely helpful in categorizing chemical clusters literally ranging from a single to an infinite number of skeletal elements of the main group and transition metals. The method involves decomposing a cluster formula comprising of a number of skeletal elements using skeletal numbers into a single whole number (K). Knowing the number of skeletal elements in a cluster (n), the K(n) parameter is obtained. The type of series S=4n+q is readily derived from the K(n) parameter. This is followed by the derivation of the capping parameter, Kp=C^yC[Mx] and the categorization parameter K*=C^y+D^z where y+z=n, the number of skeletal elements in the cluster. The cluster valence electrons can be calculated from the relationship VE=12y+14z+2 for transition metal clusters or VE=2y+4z+2 for main group elements or VE=2y+4z+2+10m where m=the number of transition metal elements present when a cluster has a mixture of elements from the main group and transition metals. The categorization parameter can also act as a guide to predict a possible symmetry of a cluster and to construct an isomeric skeletal shape of a cluster. This approach was used to categorize more than 20 metalloborane clusters as well as a few non-metalloborane clusters for comparison purposes. The isomeric skeletal structures so constructed obey the 8 or 18 electron rule as defined by coordination theory.

Keywords: Capping theory; Capping parameter; Categorization parameter; Coordination theory; Skeletal number; 4N series.

Introduction

In chemistry, some clusters have been categorized as closo, nido, arachno, hypho and klado, among others [1-7]. In this regard, the polyhedral skeletal electron pair theory (PSEPT) has been extremely useful in this process [8-11]. On closer analysis, it was observed that the transition metal clusters follow the series S=14n+q while the main group element clusters follow a parallel series S=4n+q where n represents the number of skeletal elements in a cluster and q is a numerical determinant that determines the category of the cluster. For categorization of clusters, it was found to be more convenient to utilize the S=4n+q for both main group and transition metal clusters except when the cluster valence electrons are being calculated whereby S=14n+q is applied for transition metal clusters [3,4]. The skeletal numbers which were derived from the series have been found to be extremely useful in the derivation of chemical graph theory and equations for calculating cluster valence equations [6]. In addition, they can be used as a guide to predict the possible geometrical and graphical structures of clusters [6]. Furthermore, skeletal numbers can be regarded as individual contributions to the skeletal cluster number parameter K(n) by the elements working together as a ‘team’ in the cluster. The 4N series approach was earlier applied to categorize metalloboranes. In this paper, the application of skeletal numbers to categorize metalloboranes will be demonstrated.

Results and Discussions

The application of the skeletal number to categorizing clusters is a new concept [5] and the K(n) parameter is usually a whole number. Since this approach is new, the skeletal numbers have been reproduced in table 1.

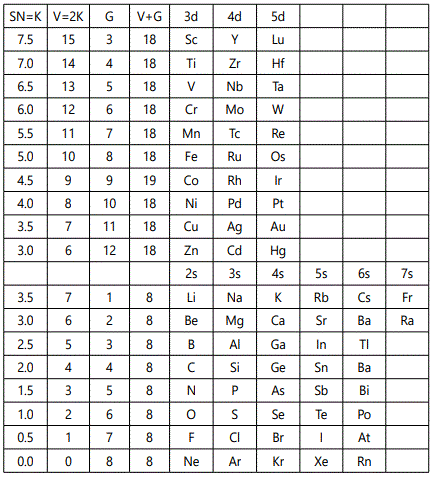
Table 1. The skeletal numbers of the main group and transition element
SN: Skeletal Number; V: Valence; G: Group valence electrons.

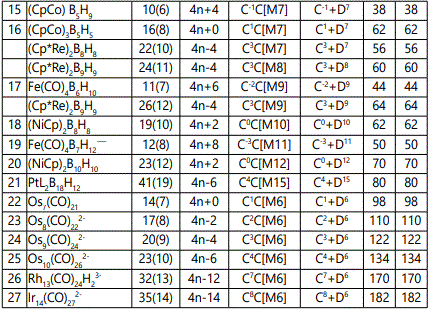
The Condensed outline of the Method for categorization of Clusters

The first step in cluster categorization is to convert the cluster formula into a number. This approach focuses on the compounds or clusters from the main group or transition metals. This is now possible since each of the periodic group of elements was assigned a skeletal number as well as the ligands. The determination of the K value for a ligand is simple; LIGANDS: FOR EVERY 1 ELECTRON DONATED, K=-0.5 AND FOR EVERY 1 ELECTRON REMOVED, K=+0.5. So the cluster number K is readily calculated for any cluster with or without ligands. This is summarized in Scheme 1 and illustrated by in the following examples and worked out exercises most of them with detailed explanations.

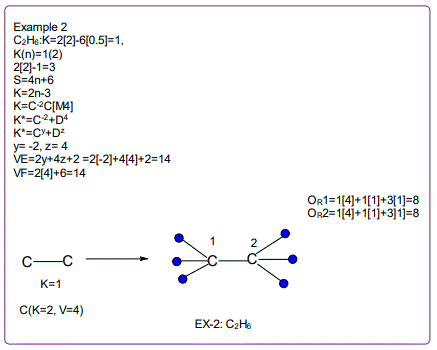
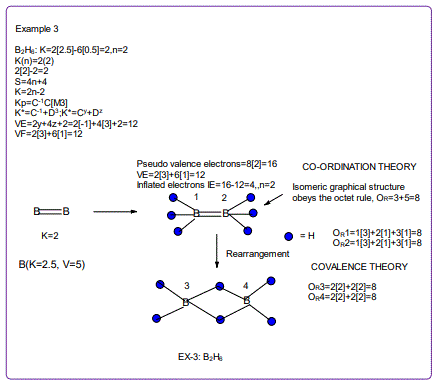
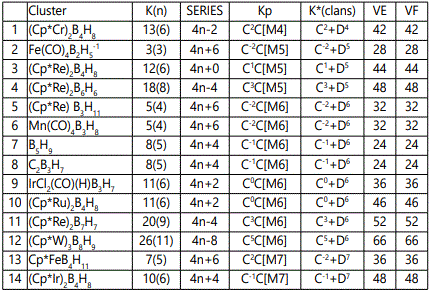
In the examples 1-7, the use of skeletal numbers to categorize clusters has been highlighted. A cluster is categorized into a CLAN series D^z and the family series C^y via the categorization parameter K*= =C^y+D^z. The same parameter can be used to calculate the cluster valence electrons VE=12y+14z+2 for transition metals and VE=2y+4z+2 for main group elements. The same parameter can be used as a guide to predict the shape of the cluster. For instance, K*=C⁰+D⁶, is an octahedral symmetry, K*=C¹+D⁶ is a mono-capped octahedron, K*=C²+D⁶; bi-capped octahedron, K*=C³+D⁶; is a tri-capped octahedron and so on. Also a list of worked out examples mainly metabollanes from 1 to 22 were analyzed and categorized into clans and families and are given in table 2. Their graphical skeletal structures constructed according to skeletal valence principle: while they may involve inflated cluster valence electrons, they generate the correct cluster formulas, they act as a guide for predicting possible structure of the cluster, and above all, the capping cluster formula which is so accurate in determining cluster valence electrons, is based on the same principles.

Scheme 1. Derivation of the series formula and categorizing parameter.

Coordination Theory

According to the 4N series approach to clusters it makes understanding much easier if we regard each linkage line to a skeletal element(node) as donating 1 electron to the nodal point. In that way, methane, CH₄ can be explained as the central carbon atom which has its own 4 electrons receiving donations from the 4H atoms in order to attain the 8-electron configuration. Each of the 4 hydrogen ligands has donated 1 electron to the node and the octet rule has been obeyed. This is the way coordination theory is viewed according to the 4N series approach. This is illustrated in EX-1A. However if we consider BH5, according to the 4N series approach, the node(B) has 3 valence electrons and the addition of 5 electrons to the node will give the node a total of 8 electrons enabling the node to achieve the eighteen electron rule. In fact, the cluster BH5 appears in a disguised form as BH₄—.According to the4N series method, there is no difference between a charge of -ve one and 1H ligand donor.

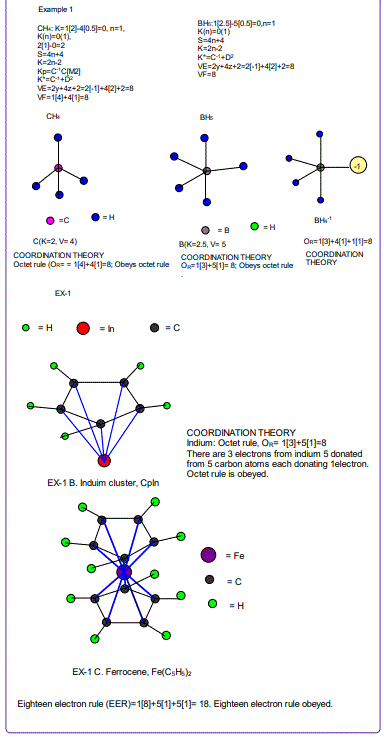
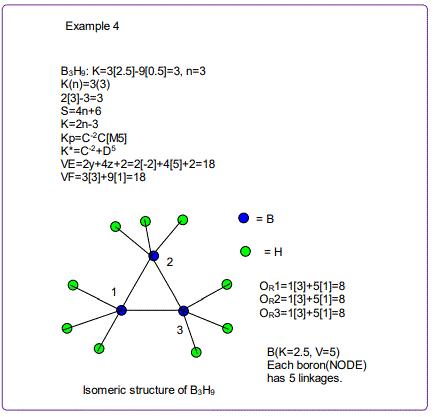
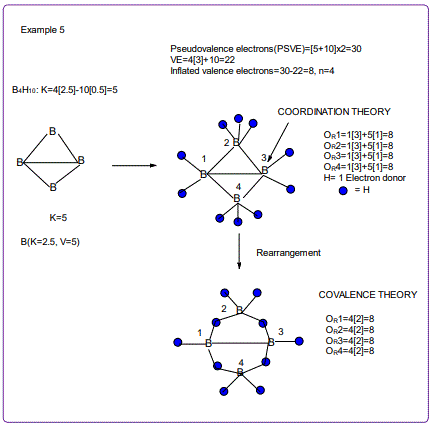
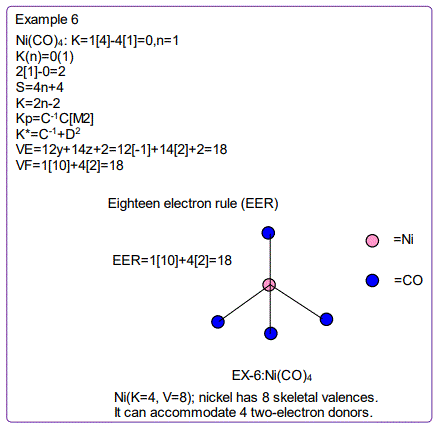
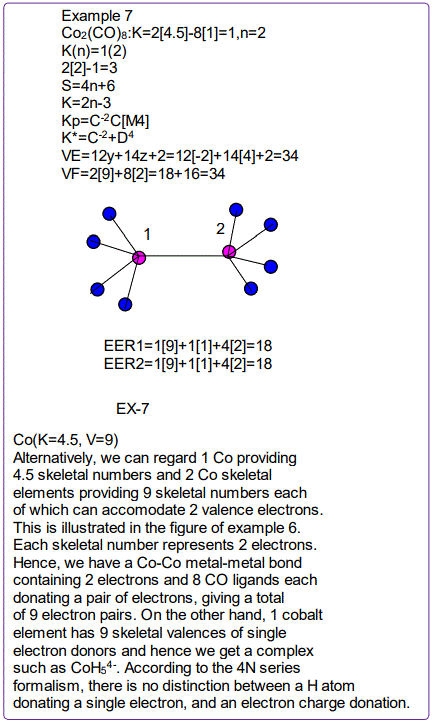
Examples 1-7: The use of skeletal numbers to categorize clusters.

All the isomeric graphical structures are constructed in line with coordination theory and they obey the octet rule for main group elements or eighteen electron rules for transition metals.

Categorization of Clusters into Clans and Families

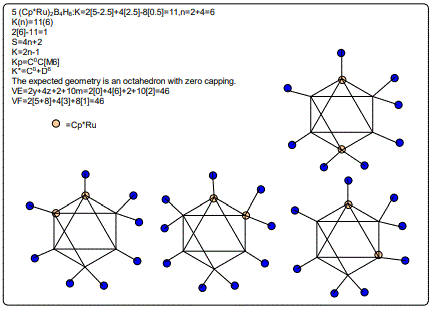
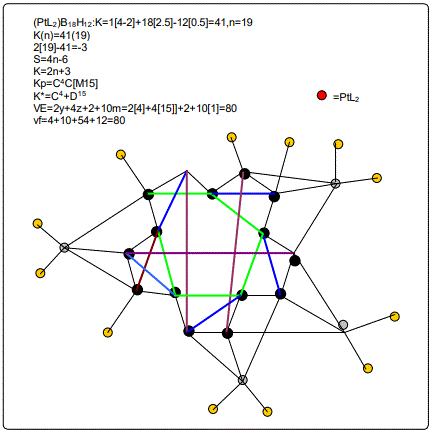
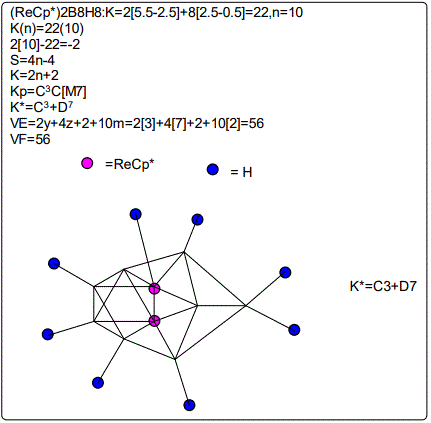
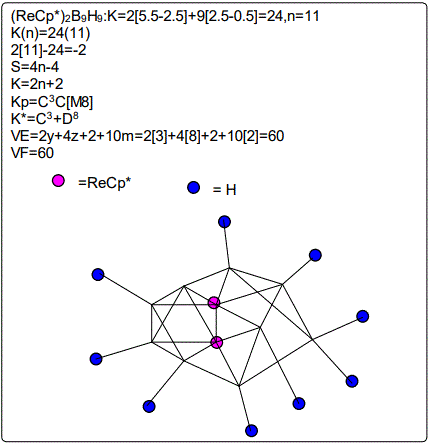
The sample of clusters mainly metaboranes were categorized into clans (D^z) and families (C^y). As can be seen from table 2, they range from D⁴ to D¹⁵ and with families from C^-2 to C⁵. The following clan series of the metalloboranes were identified, D⁴(1), D⁵(3), D⁶(8), D⁷(4), D⁹(1), D¹⁰(1), D¹¹(1), D¹²(1) and D¹⁵(1). Also some D⁶ samples were added for comparative purposes. They belong to the family series C¹, C², C³, C⁴, C⁷ and C⁸ of the D⁶ clan. The isomeric skeletal shape is also provided with explanation for each of the clusters analyzed and categorized. The corresponding isomeric skeletal structures are from figures 1-24. The categorization symbol K*=C^y+D^zcan be viewed as representing a nucleus, D^z and the outer shell C^y. The valence electrons of the cluster comes from the nuclear component which follow the VE1=S=14z+2 while the capping electrons are given by VE2=12y giving the total valence electrons VE=VE2+VE1=12y+14z+2.

The added D⁶ clan series have families ranging from C¹ to C⁸. The isomeric graphical skeletal structures of clusters adopted in this paper are constructed based on the simple coordination theory as defined in this work. Once such a structure has been constructed, the original cluster formula usually emerges from it. Furthermore, each node obeys the 8-electron or 18-electron rule if it is from the main group or transition metals respectively. Each of the linkage in the cluster represents a 1-electron donor to the node (vertex). Each node is linked to the number of lines in accordance with the skeletal valence of the fragment at the vertex. The decomposition of a cluster formula into a number followed by the categorization of the cluster into clan and family type (K*=C^y+D^z) is well explained in each of the 22 cluster examples selected.

Figure 1. Isomeric graphical shape of B₅H₉. The skeletal numbers apart from being used to predict the shape of the skeletal elements, can also be used as a guide to sketch the tentative ligand distributions onto the skeletal elements. Since the ligands carry negative skeletal numbers, their addition to a skeletal node should result in the node having the net K value of zero. That is, the node has attained either the 8 or 18 electron rule. The K values of the nodes reflect the number of ligands needed to neutralize the K value of the node to zero.

Figure 2. Figure 2. Isomeric structures of B Isomeric structures of B₄H₄CoCp. In this example, skeletal numbers have been applied as a guide to determine the possible distributions of the ligands at every node. Alternatively, if the skeletal valence of each node is known, then the ligands are readily added to the node. In this case, CoCp^*(K=4.5-2.5=2. V=4). This means the fragment has 4 linkages while boron node B K=2.5, V=5 will have 5 linkages. There are 3 linkages already at the base. For the boron node the base needs 2 linkages to satisfy the valence rule and for the CoCp^* fragment of valence 4, only one linkage to be added on is needed.

Figure 3. Isomeric structures of C₂B₃H₇. Skeletal numbers have been applied to the skeletal elements. Also the skeletal valences at each node could be used. For the skeletal elements boron, K=2.5, V=5. That means each boron node has 5 linkages while carbon with K=2, V=4 has 4 linkages. With this information, the hydrogen atoms can easily be assigned to the skeletal atoms.

Figure 4. Isomeric skeletal structure of (Cp^*Ir)₂B₄H₈. The skeletal valence rule can assist us distribute the ligands onto the node points. This is easier than using skeletal numbers as in the examples discussed earlier. At the Cp^*Ir nodal point, the valence is 4 and hence there will be 4 linkages. On the other hand at the boron(B) node, the skeletal valence is 5, and so there will be 5 linkages.

Figure 5. Isomeric skeletal structures of (Cp^*Ru)₂B₄H₈. Ligand distribution follows the skeletal valence rule. For instance, RuCp^*(K=5-2.5=2.5, V=2K=5), and B(K=2.5, V=5). This means that both RuCp^* and B will each have 5 linkages. Thus RuCp^* is equivalent to B in terms of connectivities. When the valence rule is followed, the skeletal elements and the ligands fit so well as to generate the parent cluster formula.

Figure 6. The graphical isomeric skeletal structure of (RuCp^*)₂B₄H₈. The skeletal valence= 5 and each fragment will have 5 linkages at each node. This allows us to distribute the ligands accordingly. This is shown in figure 6.

Figure 7. Isomeric skeletal structure of (Cp^*Cr)₂B₄H₈. Accordingly to the capping series, D⁴ follows the S=4n+2 family of clusters. Hence, its linkages are given by K=2n-2=2[4]-1=7. That is, it is a tetrahedron linked by 7 linkages instead of the normal 6 linkages. The double capping will then be superimposed onto the 7 linked tetrahedral shape. The fragment Cp^*Cr(K=6-2.5=3,5, V=7). The fragment will possess 7 skeletal valences. This is reflected in thefigure 7. The fragment Cp^*Cr(K=3.5, V=7) has a valence of 7. Hence, it will have seven connectivities around it. Likewise, boron will also have 5 connectivities.

Figure 8. Isomeric skeletal structure of (WCp^*)₃B₈H₉. The fragment WCp^*(K=6-2.5=3.5, V=7) has a skeletal valence of 7. Hence, it will have 7 connectivities around it whereas boron will have 5 connectivities since its skeletal number is 2.5.

Figure 9. Isomeric skeletal structure of Mn(CO)₄B₃H₈. The fragment Mn(CO)₄(K=5.5-4=1.5, V=3). This means it will have 3 linkages to it. The boron will portray its usual connectivity of 5.

Figure 10. Isomeric structure of (Cp^*Re)₂B₄H₈. The fragment ReCp^*(K=5.5-2.5=3, V=6) has a valence of 6. Hence, it will have 6 linkages around it. The boron element will have the usual 5 skeletal linkages.

Figure 11. Isomeric skeletal structure of Gp^*ReB₃H₁₁. The fragment ReCp^*(K=5.5-2.5=3, V=6) has a skeletal valence of 6. These are taken up by the 3 linkages and 3 hydrogen atoms. Boron has 5 linkages.

Figure 12. Isomeric skeletal structure of (Cp^*Fe)B₄H₁₁.

Figure 13. Isomeric skeletal structure of (ReCp^*)₂B₆H₆.

Figure 14. Isomeric skeletal structure of (Cp^*Re)₂B₇H₇.

Figure 15. Isomeric skeletal structure of Fe(CO)₄B₆H₁₀.

Figure 16. Isomeric skeletal structure of (CpCo)₃B₅H₅.

Figure 17. isomeric skeletal structure of FeB₇H₁₂(CO₄)^-1.

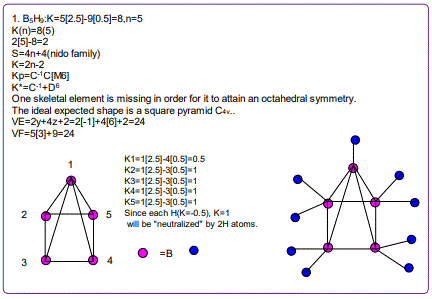
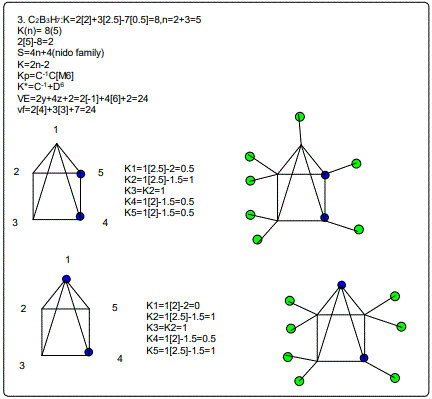
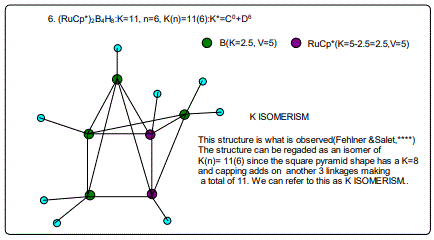
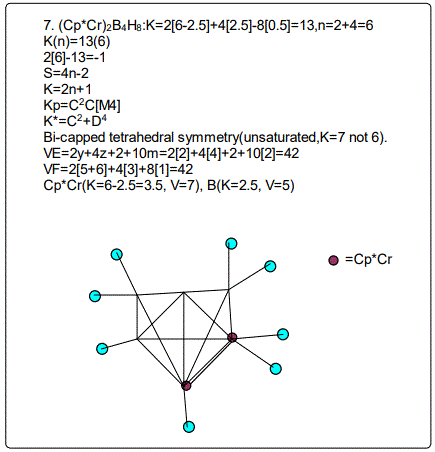
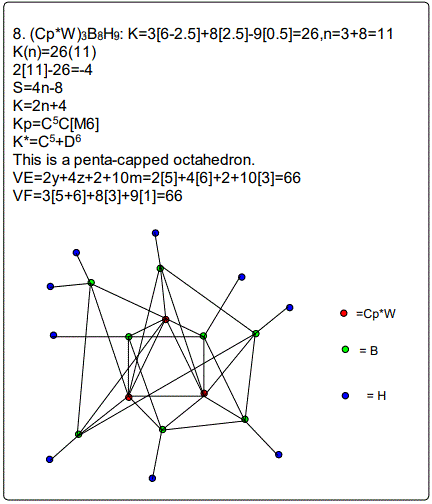
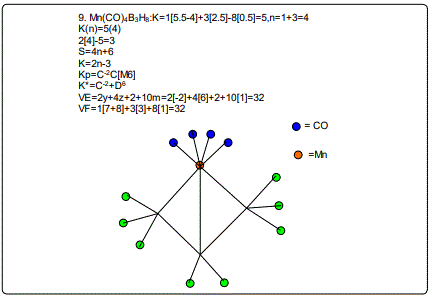
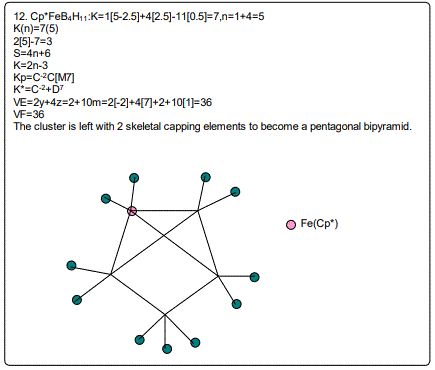
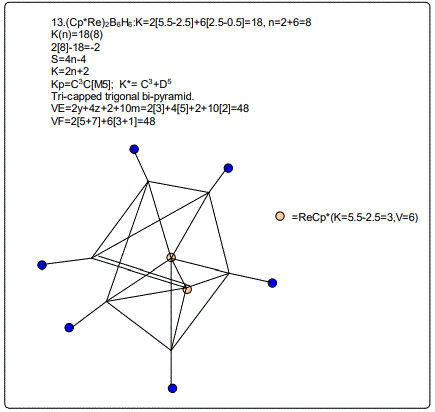
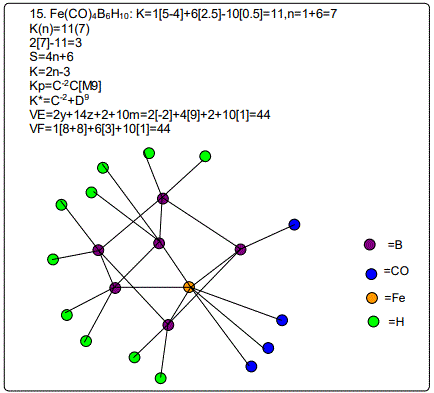
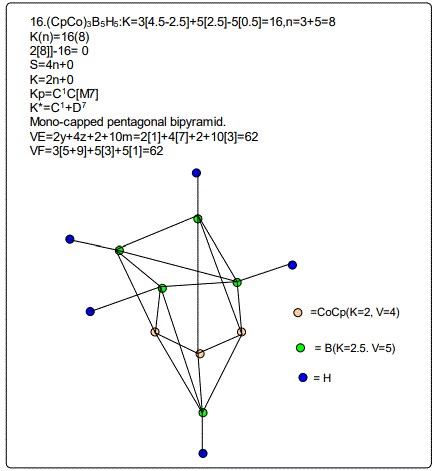
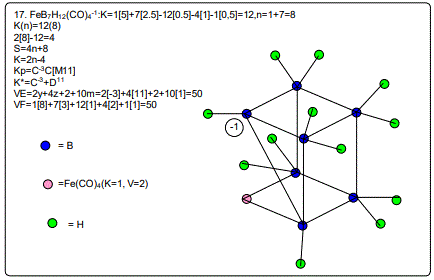
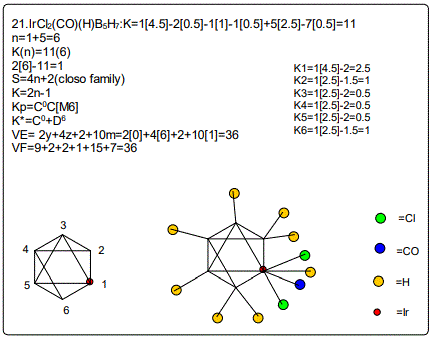
18. (CpCo)B₅H₉:K=1[4.5-2.5]+5[2.5]-9[0.5]=10,n=1+5=6
K(n)=10(6)
2[6]-10=2
S=4n+4(nido)
K=2n-2
Kp=C^-1C[M7]
K*=C^-1+D⁷
VE=2y+4n+2+10m=2[-1]+4[7]+2+10[1]=38
VF=1[5+9]+5[3]+9[1]=38
The isomeric structure has not been provided as it can be generated in the same procedures as above ones.

Figure 18. Isomeric skeletal structure of (NiCp)₂B₈H₈.

Figure 19. Isomeric graphic structure for (NiCp)₂B₁₀H₁₀.

Figure 20. Isomeric skeletal structure of Fe(CO)₄B₂H₅^-1.

Figure 21. Isomeric skeletal structure of IrCl₂(CO)(H)B₅H₇.

Figure 22. Isomeric graphical structure of (PtL₂)B₁₈H₁₂.

Figure 23. Isomeric skeletal structure of (ReCp^*)₂B₈H₈.

Figure 24. Isomeric skeletal structure of (ReCp^*)₂B₉H₉.

Table 2. A collection of selected metaborane clusters.

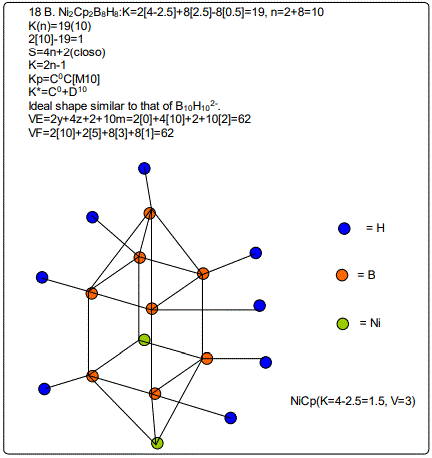
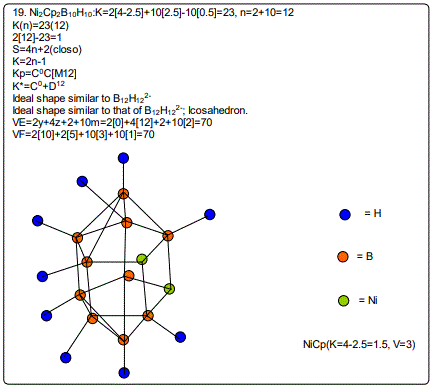
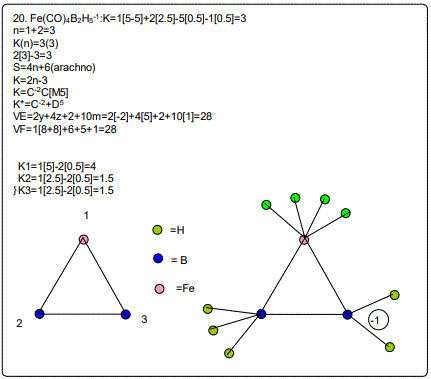
Derivation of Information from the Categorization Parameter K*=C^Y+D^Z

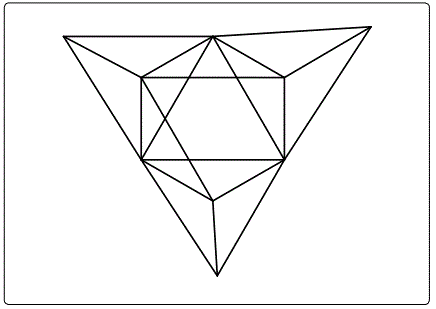
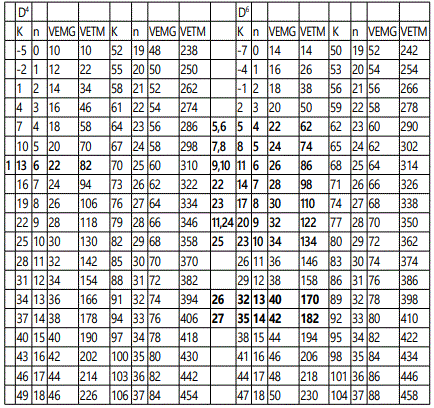
Once a cluster formula has been converted into a categorization parameter K*=C^y+D^z all the important information is concealed into that symbol. All that is needed to know is whether it refers to the main group or transition metals. For instance, K*=C³+D⁶; refers to a cluster with 9 skeletal elements (n=3+6=9), VE=12y+14z+2=12[3]+14[6]+2=122 (transition metals) and VE=2y+4z+2=2[3]+4[6]+2=32 (main group elements). The ideal shape will be a tri-capped octahedron as shown in figure 25. The clan series do generate an infinite range of K(n) and VE series. The numerical series from n=0 to n=37 for D⁴ and D⁶ for both main group and transition metals are given in table 3. In order to highlight the significance of categorization of clusters into clans, a small sample of D⁵ series was categorized and is given in the examples G1 to G6. The final categorization results of the D⁵ series are: K*=C⁵+D⁵; C⁹+D⁵; C¹⁰+D⁵; C¹¹+D⁵; C¹²+D⁵; and C¹⁷+D⁵. Also selected clan series of D⁵ are given in table 4. The positions of the K(n) and VE values of G1 to G6 clusters are highlighted in table 4. The D⁵ and D⁶ represent clan clusters whose structures are centered around an ideal trigonal bipyramid and octahedral nuclei respectively. The ideal structures are shown in figure 26.

Figure 25. An ideal isomeric structure of a tri-capped octahedral symmetry.

Figure 26. Ideal symmetries of trigonal bipyramid and octahedral nuclei.

The variation of the skeletal number in similar cluster formulas

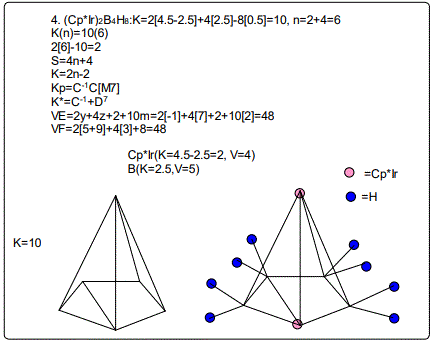
Let us focus on the case of the following clusters (Cp^*M)₂B₄H₈, M=Ir, Ru, Re and Cr as an illustration of the concept. The corresponding K(n) parameter and categorization parameter K* are as follows;10(6),C^-1+D⁷; 11(6),C⁰+D⁶; 12(6),C¹+D⁵;13(6),C²+D⁴. The K values for Ir, Ru, Re, and Cr are 4.5, 5.0, 5.5 and 6 respectively the K(n) parameter starting with K=10 to 11, 12 and then 13 for the same number of skeletal elements n=6. As can be seen, the number of skeletal elements remains the same while the value of the cluster number K increases. This results into the increase in the capping index while the nuclear index is decreasing. In the parameter K*=C^y+D^z and n=y+z. Since n is constant, the increase in y will naturally result in the corresponding decrease in z. Hence, the increase in the K value results in the increase of the capping index (the outer shell expands) and a decrease in the nuclear index (nucleus shrinks).

Table 3.The variation of K(n) parameter with cluster valence electrons startrom n=0 for D4 and D6 series

VEMG: Cluster Valence Electrons for Main Group Elements; VETM: Cluster Valence Electrons of Transition.

Table 4. The variation of the K(n) values starting with n=0 for D⁴ cluster series.

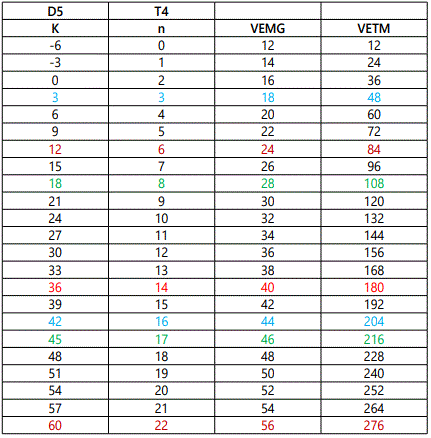
G1. F=Os₁₀(C)(CO)₂₄:K=10[5]-1[2]-24[1]=10,n=10
       K(n)=24(10)
       S=4n-8
       K=2n+4
       Kp=C⁵C[M5]
       K*=C⁵+D⁵
       K*=C^y+D^z
       VE=12y+14z+2=12[5]+14[5]+2=132
       VF=10[8]+1[4]+24[2]=132

G2. Rh₁₄(CO)₂₅^4-:K=14[4.5]-25[1]-4[0.5=36,n=14
       K(n)=36(14)
       S=4n-16
       K=2n+8
       Kp=C⁹C[M5]
       K*=C⁹+D⁵
       K*=C^y+D^z
       VE=12y+14z+2=12[9]+14[5]+2=180
       VF=14[9]+25[2]+4[1]=180

G3. Rh₁₅(CO)₂₇^3-:K=15[4.5]-27[1]-3[0.5]=39,n=15
       K(n)=39(15)
       2[15]-39=-9
       S=4n-18
       K=2n+9
       Kp=C¹⁰C[M5]
       K*=C¹⁰+D⁵
       K*=C^y+D^z
       VE=12y+14z+2=12[10]+14[5]+2=192
       VF=15[9]+27[2]+3[1]=192

G4. Pd₁₆(CO)₁₃L₉:K=16[4]-13[1]-9[1]=42,n=16
       K(n)=42(16)
       2[16]-42=-10
       S=4n-20
       K=2n+10
       Kp=C¹¹C[M5]
       K*=C¹¹+D⁵
       VE=12y+14z+2=12[11]+14[5]+2=204
       VF=16[10]+13[2]+9[2]=204

G5. Rh₁₇(CO)₃₀^3-: K=17[4.5]-30[-1]-3[0.5]=45,n=17
       K(n)=45(17)
       2[17]-45=-11
       S=4n-22
       K=2n+11
       Kp=C¹²C[M5]
       K*=C¹²+D⁵
       VE=12y+14z+2=12[12]+14[5]+2=216
       VF=17[9]+30[2]+3[1]=216

G6. Rh₂₂(CO)₃₇^4-:K=22[4.5]-37[1]-4[0.5]=60,n=22
       K(n)=60(22)
       2[22]-60=-16
       S=4n-32
       K=2n+16
       Kp=C¹⁷C[M5]
       K*=C¹⁷+D⁵
       VE=12y+14z+2=12[17]+14[5]+2=276
       VF=22[9]+37[2]+4[1]=276

Conclusion

A good range of metalloboranes have been analyzed and categorized into clans and families of clusters using skeletal numbers. The cluster valence electrons and isomeric skeletal structures can readily be derived. The metalloborane (Cp^*W)₃B₈H₉ with a categorizing parameter K*=C⁵+D⁶ was found surprisingly highly capped unlike other metalloboranes. A formula for calculating cluster valence electrons for a cluster with a mixture of non-metals was derived namely: VE=2y+4z+2+10m where y is a capping index for C^y elements and z is a capping index for D^z elements and m is the number of metal skeletal elements in the cluster. Thus in the case of the (Cp^*W)₃B₈H₉, y=5,z=6 and m=3 which gives us a cluster valence value of 66. The 10m component of the formula is for metal adjustment to take into account the additional cluster valence electrons introduced by metal atoms present.

Acknowledgement

I wish to thank Dr. Penelope Emma Kiremire for editing the draft paper.

References

Greenwood NN. Metalloboranes. Pure Appl Chem. 1983; 55(9): 1415-1430. doi: 10.1351/pac198355091415
Housecroft CE, Sharpe AG. Inorganic Chemistry. 2nd Edition. Pearson, Prentice Hall, Harlow, England. 2005.
Kiremire EM. The Application of the 4n Series Method to Categorize Metalloboranes. Int J Chem. 2016; 8(3): 62-73. doi: 10.5539/ijc.v8n3p62
Kiremire EM. Classification of Zintl Ion Clusters Using 4n Series Approach. Orient J Chem. 2016; 32(4): 1731-1738. doi: 10.13005/ojc/320401
Kiremire EM. The Outstanding Applications of Skeletal Numbers to Chemical Clusters. Int J Chem. 2017; 9(3): 28-48. doi: 10.5539/ijc.v9n3p28
Kiremire EM. The Capping Theory of Chemical Clusters Based on 12N/14N Series. Int J Chem. 2018; 10(4): 130-154. doi: 10.5539/ijc.v10n4p130
Miessler GL, Fischer PJ, Tarr DA. Inorganic Chemistry. 5th Edition, Pearson Education, Inc., Upper Saddle River. 2013.
Mingos DMP. A General Theory for Cluster and Ring Compounds of the Main Group and Transition Elements. Nature Physical Science. 1972; 236: 99-102. doi: 10.1038/physci236099a0
Wade K. The structural significance of the number of skeletal bonding electron-pairs in carboranes, the higher boranes, and borane anions and various transition metal carbonyl cluster compounds. Chem Commun. 1971; 792-793. doi: 10.1039/C29710000792
Wade A. Structural and Bonding Patterns in Cluster Chemistry. Adv Inorg Chem Radiochem. 1976; 18: 1-66. doi: 10.1016/S0065-2792(08)60027-8
Welch AJ. The significance of Wade’s rules. Chem Commun. 2013; 49: 3615-3616. doi: 10.1039/C3CC00069A

International Journal of Chemistry and Research

ISSN: 2642-1615

Search

Full Text Contents