Research Article
Finite Element Analysis of 3 and 4 Units Zirconium Fixed Partial Dentures
1Department of Prosthetic Dentistry, Faculty of Dentistry, Kocaeli University, Kocaeli, Turkey
2Private Office, Istanbul, Turkey
3Okmeydanı Dental Hospital Istanbul, Turkey
*Corresponding author: Ayse Kocak-Buyukdere, Department of Prosthetic Dentistry, Faculty of Dentistry, Kocaeli University, Yuvacık Yerkleşkesi, Turkey, Tel: +90 532 3165759, Fax: +90 262 344 2109, E-mail: akocakbuyukdere@gmail.com
Received: September 17, 2016 Accepted: September 21, 2016 Published: January 6, 2017
Citation: Kocak-Buyukdere A, Sertgoz A, Dergin C. Finite Element Analysis of 3 and 4 Units Zirconium Fixed Partial dentures. Madridge J Dent Oral Surg. 2017; 2(1): 23-27. doi: 10.18689/mjdl-1000106
Copyright: © 2017 The Author(s). This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Abstract
Introduction: Several studies have used experimental, analytical, and computational models by means of finite element models (FEM), photo elasticity, strain gauges and associations of these methods to evaluate the biomechanical behavior of dental ceramics.
Finite element analysis of complex structures used in engineering applications is a method for making the analysis in computer environment by converting to digital models clinical conditions is achieved by using virtual models. The resulting model is intended to solve the problem mathematically divided into a certain number of smaller.
The aim of the study is, to determine the stress distribution at 3 or 4 units zirconium fixed partial dentures having 3 differences connector thickness under occlusal forces in finite element method.
Material and Methods: Finite element analysis: a three-dimensional model of a three-unit and four-unit posterior bridges with 3 different designs were created on Pentium IV, 2.10 GHz, 2×512 RAM computer platform by means of the solid modelling program of the finite element software I-DEAS (Master Series 11.0, Structural Dynamics Research Corporation, Milford, Ohio).
Results: Maximum “Von Mises” stress values were formed in different values in different zirconium based fixed partial dentures. The maximum stress were occurred in 3 unit zirconium based fixed partial dentures as 754MPa, for the 4 units zirconium based fixed partial is 926MPa. There is no differences in all connectors in both 3 unit and 4 unit zirconium based fixed partial dentures.
Conclusion: Results showed that stress values were not differed for the finite element models having different connector thickness. However, stress values increased for the 4units zirconium restorations than the 3 units.
Keywords: Finite Element Test; zirconium Bridges.
Introduction
The unique properties of dental materials, including the tooth structure durability, toughness, esthetic, biocompatiblity, same abrasion effect as natural teeth, similar thermal expansion as enamel, low thermal conductivity, not hypersensitive, low cost, product easily [1-4].
The core ceramic is commonly composed of crystalline nepheline or lithium disilicate in a glass matrix, or zirconium oxide. Zirconium oxide was introduced as core material for all-ceramic restorations because of its good chemical and dimensional stability, high mechanical strength and toughness [1]. The veneer consists of a glass and a crystalline phase of fluoroapatite, aluminum oxide or leucite [1]. Veneers are the weakest part of the restorations because of their non homogenous structure [5]. Chipping of veneering porcelain from the surface of framework occurred [6,7].
Veneer and the core materials connections are most important part of the restorations. Fractures mostly occurs between that connections while chewing [2,5].
One of the main failure of the restorations are cracks, which are occured within thin ceramic layers at the lower crown cementation surface beneath the contact [7].
Dental bridges cannot be produced under ideal conditions [8]. And also all dental bridges are individual so that it is difficult to have standartization [8].
To predict stresses that develop in the structures under load with the purpose of examination made using some tools called stress analysis [9-11]. Photo elastic stress analysis, stress analysis is performed with tension gauges, fragile varnish stress analysis, laser beam stress analysis, finite element stress analysis methods are the different stress analysis [9-14].
Finite element method (FEM) studies reflect an ‘ideal’ condition of the component to be analyzed [8]. Two-dimensional and three-dimensional analysis method can be used in FEM. Two-dimensional finite element analysis method may be inadequate due to the complexity of the structure in most studies [15,16]. However, two-dimensional finite element analysis is used for the purpose of preliminary assessment [17]. Three-dimensional finite element analysis is preferred in more complex and requires engineering knowledge [18,19]. First of all geometric model is created for FEM. Then the initial and boundary conditions of the system are determined that affect the analysis results. Poissonʼs ratio of the elements defined in the model and modulus of elasticity values are determined [20].
Dental bridges cannot be produced in an industrial surrounding under ideal conditions like stems for the total hip replacement or mechanical heart valves, for example. Therefore, it is of importance in addition to the FE analyses to perform experimental tests [8,13,21,22].
FEM, basically a mathematical modeling technique used to determine the general characteristics of a structure. FEM was first introduced, which principle of working is from “pieces to all”, in 1950 in aerospace engineering. In 1970, progress in FEM mechanical, electrical, construction, hydrodynamics engineering fields and also medicine, dental medicine were used it [23,24]. Mainly based on the separation of the structural components, the method is defined as the finite element. Geometric objects are divided into elements which can be easily calculated on a computer. To have a more sensitive measurement for power distribution using a large number of elements is important [18,23]. To determine stress in an asymmetric pattern following information is needed [25]. The number of joint, the number of elements, the numbering system for determining each element, the Youngʼs modulus and Poissonʼs ratio of each element, the coordinates of each node, type of boundary conditions, determination of applied load [8].
The purpose of this study was to analyze the stress levels in the structures of an two different fixed partial dentures with different connectors length.
Materials and Methods
Finite element analysis: a three-dimensional model of a three-unit and four-unit posterior bridges with 3 different designs were created on Pentium IV, 2.10 GHz, 2×512 RAM computer platform by means of the solid modeling program of the finite element software I-DEAS (Master Series 11.0, Structural Dynamics Research Corporation, Milford, Ohio).
3 unit zirconium bridge with one premolar (Figures 1 and 2) and 4 units zirconium bridges with one premolar and molar (Figures 3 and 4) with 3 different connection thickness were analysed by the finite stress analyses test. Solid modelling, dental bridges mesh geometry, plate elements and nodes and boundary elements are the four different part of the study.
3 and 4 unit zirconium bridge restorations were designed for 3 different connection thickness.
a) Model I: 3 unit fixed partial dentures- 9mm2
crosssectional area
b) Model II: 3 unit fixed partial dentures- 12mm2
crosssectional area
c) Model III: 3 unit fixed partial dentures- 15mm2
crosssectional area
d) Model IV: 4 unit fixed partial dentures- 9mm2
crosssectional area
e) Model V: 4 unit fixed partial dentures- 12mm2
crosssectional area
f) Model VI: 4 unit fixed partial dentures- 15mm2
crosssectional area

Solid modelling technique is used and models are produced “quadratic tetrahedral” structures. All materials were assumed as homogenous, isotropic, and linearly elastic.
The three-dimensional models for three-unit posterior bridges were meshed with approximately 39652 and 25067 cardinality and also 19421, 11747 meshes were used for four units bridges.
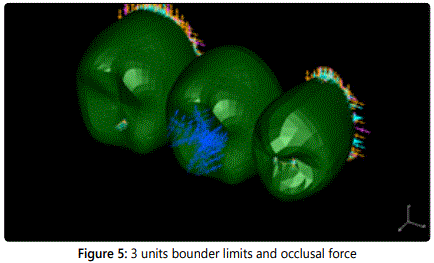
Occlusal forces position, direction and meshes unrestricted limits of meshes were analyzed in that part of the study. 300 N forces were applied on the pontics occlusal plates (Figure 5). The same forces were dizayned for the two pontics for the four units bridges (Figure 6).
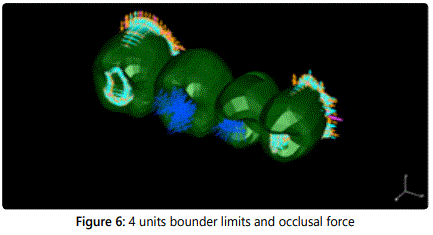
Zirconium bridges internal surfaces meshes were dizayned as stable on X Y Z axis. On the other surfaces meshes were unrestricted in 3 dimensional position.
All materials are expected as homogenous and isotropic which are used in analyzed 1 material properties used in analysis were taken from the literature, elastic modolous of zirconium (CERCON Smart) is 210Mpa, possionʼs ratio 0,300 elastic modulous of porcelain (CERCON Ceram S) elastic moduolus is 60Mpa possionʼs ratio is 0.265 ve Zirkon (CERCON Smart) elastik modül 210MPa, possionʼs oranı 0.300 Porselen (CERCON Ceram S) elastik modül 60MPa, possionʼs oranı 0.265 verilmiştir.
Boundary conditions and network structures determined using a finite element model created engineering software program (I-DEAS; Master Series 11.0, Structural Dynamics Research Corporation, Milford, Ohio) in Pentium IV, 2.10 GHz, 2×512 RAM.
Results
Boundary conditions set and the network structure created finite element models I-DEAS; Master Series 11.0, using engineering (Structural Dynamics Research Corporation, Milford, Ohio) in Pentium IV, 2.10 GHz, 2×512 RAM analysis were done. Maximum von Mises stress values that resulted both 300 N static occlusal loading with different connectors numbers and thickness are illustrated in table 1.
Maximum “Von Mises” stress values were formed in different values in different zirconium based fixed partial dentures. The high stress were occurred in 3 unit zirconium based fixed partial dentures as 754 MPa (Figure 7-9), for the 4 units zirconium based fixed partial is 926MPa (Figure 10-12). There is no differences in all connectors in both 3 unit and 4 unit zirconium based fixed partial dentures.
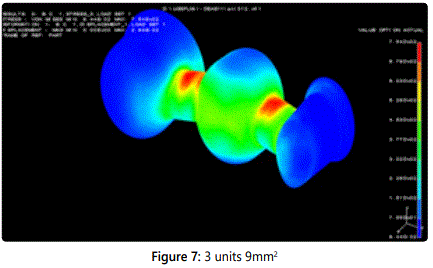

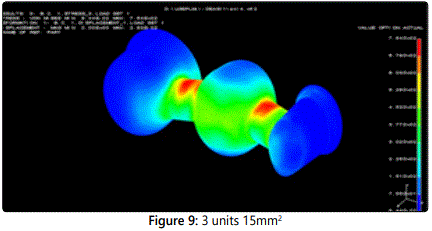

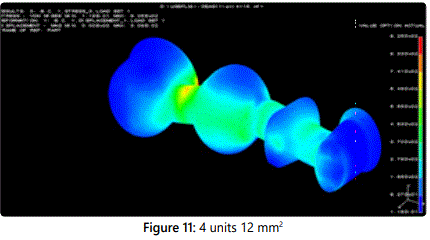
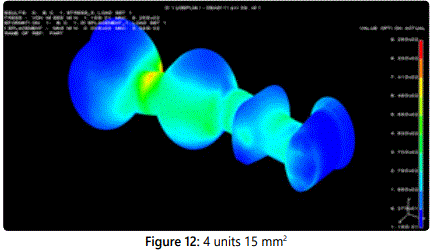
Maximum stress were occurred in connectors which are between teeth and the pontics. In addition, stress were seen in the 4 unit bridges in the marginal region of the abutment teethʼs connector side.
Discussion
During the function of the dental restorations to withstand occlusal forces, is an important for the longevity of the restoration. Occlusal forces determined difference in the studies, while biting occlusal forces 263 N, swallowing time it is reported 297 N. The highest chewing forces is in molar regions is 400-800 N; and also reported occlusal forces ain premolar region 220-450 N; canine region 130-330 N and incisors 90-150 N [26].
Apholt et al reported the mechanical properties, fixed-partial dentures require 400 N for the anterior and 600 N for the posterior region [27]. In our study 300 N is used.
Connection design is important for long term success of the zirconium fixed partial restorations. In order to withstand the occlusal forces connectors have enough thickness, rounded corners and avoid from sharp edges [28]. In the studies, fractures are mostly seen in connectors [27,29]. Also in our study maximum stress were seen in the connectors.
Zirconium fused porcelain restorations have high strength. This is gained by the sintering [30]. In our study also stress are less than the withstand of the material.
Three-dimensional finite element stress analysis is a technique adopted for the solution of engineering problems. Finite element analysis of the stress analysis of the methods used to examine reactions that occur against the load in any structure; complex structures can be designed, ideal conditions could be designed [25]. In our study 3 unit and 4 unit bridges were designed in ideal conditions.
The three-dimensional finite element stress analysis has revealed that there is a superior technique. But used in this technique and material properties required for analysis, or the measured values with a number of test methods, or are mean values from the literature. From this point of stress values not quantitatively be evaluated qualitatively [31].
Finite elements used in our study are given in linear elastic stress analysis features to all models. The proportional load being applied to the deformation of the structure, but is meant to be independent of the rate of deformation. That means under the forces that has been applied to the material, the plastic deformation means display elastic properties.
In stress analyzes studies have found connectors are the most effected area in difference materials, difference bridges length [8,9,32-34].
Oh and et al. reported that gingival embrasure thickness effect the fracture of the bridges [34]. In our study when the gingival thickness did not effect the tensile forces. It can be because of the high strength of the zirconium.
In our study 3 and 4 unit dental restorations maksimum stress do not different. Maksimum stress in zirconium bridges close to the 900 MPa,which is crushing strength. That means there is a high risk for posterior region 4 unit bridges to be longivty. But 3 unit zirconium fused to bridges for the posterior region have no risk.
Conclusion
Finite element analyzes show that 3 and 4 units zirconıum bridges with different connector thickness do not affect maksimum stress and toughness. 4 unit bridges have more stress than the 3 unit bridges
Conflicts of Interest: The authors declare no conflicts of interest with this submission.
Acknowledgement
Abstract that was accepted by 18th TDA 2011 conference in İstanbul, Turkey.
References
- Aboushelib MN, de Jager N, Kleverlaan CJ, Feilzer AJ. Microtensile bond strength of different components of core veneered all-ceramic restorations. Dent Mater. 2005; 21(10): 984-91. doi: 10.1016/j.dental.2005.03.013
- Pesqueira AA, Goiato MC, Filho HG, et al. Use of stress analysis methods to evaluate the biomechanics of oral rehabilitation with implants. J Oral Implantol. 2014; 40(2): 217-28. doi: 10.1563/AAID-JOI-D-11-00066
- Su N, Liao Y, Zhang H, et al. Effects of core-to-dentin thickness ratio on the biaxial flexural strength, reliability, and fracture mode of bilayered materials of zirconia core (Y-TZP) and veneer indirect composite resins. J Prosthet Dent. 2016; 23: pii: S0022-3913(16)30138-X. doi: 10.1016/j. prosdent.2016.04.017
- Hatta M, Shinya A, Yokoyama D, Gomi H, Vallittu PK, Shinya A. The effect of surface treatment on bond strength of layering porcelain and hybrid composite bonded to zirconium dioxide ceramics. J Prosth Res. 2011; 55(3): 146–153. doi: 10.1016/j.jpor.2010.10.005
- Taskonak B, Yana J, Mecholsky Jr JJ, Sertgöz AK. A Fractographic analyses of zirconia-based fixed partial dentures. Dent Mat. 2008; 24(8): 1077– 1082. doi: 10.1016/j.dental.2007.12.006
- Fanuscu MI, Vu HV, Poncelet B. Implant biomechanics in grafted sinus: A finite element analysis. J Oral Implantol. 2004; 30(2): 59–68. doi: 10.1563/0.674.1
- Geng JP, Tan KBC, Liu GR. Application of finite element analysis in implant dentistry: A review of the literature. J Prosthet Dent. 2001; 85(6): 585–598. doi: 10.1067/mpr.2001.115251
- Darbar UR, Huggett R, Harrison A. Stress analysis techniques in complete dentures. J Dent. 1994; 22(5): 259-262. doi: 10.1016/0300-5712(94)90054-X
- Yang HS, Lang LA, Molina A, Felton DA. The effects of dowel design and load direction on dowel-and-core restorations. J Prosthet Dent. 2001; 85(6): 558-67. doi: 10.1067/mpr.2001.115504
- Desai SR, Singh R, Karthikeyan I. 2D FEA of evaluation of micro movements and stresses at bone-implant interface in immediately loaded tapered implants in the posterior maxilla. J Indian Society of Periodontology. 2013; 17(5): 637-643. doi: 10.4103/0972-124X.119283
- Kim S, Choi H, Woo D, et al. A three-dimensional finite element analysis of short dental implants in the posterior maxilla. Int J oral maxillofac implants. 2014; 29(2): e155-64. doi: 10.11607/jomi.3210.
- Almeida EO, Rocha EP, Freitas Júnior AC, et al. Tilted and short implants supporting fixed prosthesis in an atrophic maxilla:a 3d-FEA biomechanical evaluation. Clin Implant Dent Relat Res. 2015; 17: e332-e342. doi: 10.1111/cid.12129.
- Geng JP, Keson BCT, Liv GR. Application of Finite Element Analysis in Implant Dentistry: A Review of the Literature. J Prosthet Dent. 2001; 85(6): 585–598. doi: 10.1067/mpr.2001.115251
- Yang HS, Lang LA, Felton DA. Finite element stress analysis on the effect of splinting in fixed partial dentures. J Prosthet Dent. 1999; 81(6): 721-8. doi: 10.1016/S0022-3913(99)70113-7
- Hasegawa A, Shinya A, Nakasone Y, Lassila LVJ, Vallittu PK, Shinya A. Development of 3D CAD/FEM analysis system for natural teeth and jaw bone constructed from X-Ray CT images. Int J Biomater. 2010; pii: 659802. doi: 10.1155/2010/659802.
- Alkan I, Sertgöz A, Ekici B. Influence of occlusal forces on stress distribution in preloaded dental implant screws. J Prosthet Dent. 2004; 91(4): 319-25. doi: 10.1016/S0022391304000332
- Sertgöz A. Implant-supported in the crown- bridge restoration Implant Size and distal extension quantity of bone - implant Interface Three Dimensional Finite Effects of Stress Distribution Element Analysis with Stress Analysis. Marmara Univercity Health Sciences Institute Doctoral Thesis, İstanbul, 1993.
- Rasouli GAA, Geramy A, Yaghobee S, et al. Evaluation of Platform Switching on Crestal Bone Stress in Tapered and Cylindirical İmplants: A Finite Element Analyses. J Int Acad Periodontol. 2015; 17(1): 2-13.
- Apholt W, Bindl A, Lüthy H, Mörmann WH. Flexural strength of Cerec 2 machined and jointed InCeram-Alumina and InCeram-Zirconia bars. Dent Mat. 2001; 17(3): 260-7.
- Lüthy H, Filser F, Loeffel O, Schumacher M, Gauckler LJ, Hammerle CHF. Strength and reliability of four-unit all-ceramic posterior bridges. Dent Mater. 2005; 21(10): 930–37. doi: 10.1016/j.dental.2004.11.012
- Derand P, Derand T. Bond Strength of Luting Cements to Zirconium Oxide Ceramics. Int J Prosthodont. 2000: 13(2): 131-135.
- Fischer H, Maier HR, Marx R. Improved reliability of leucite reinforced glass by ion exchange. Dent Mater. 2000; 16(2): 120–128.
- Fischer H, Weber M, Marx R. Lifetime Prediction of All-ceramic Bridges by Computational Methods. J Dent Res. 2003; 82(3): 238-242.
- Oh W, Götzen N, Anusavice KJ. Infuence of connnector design fracture probability of ceramic fixed partial dentures. J Dent Res. 2002; 81(9): 623-627.


