Research Article
Common fixed point theorem in intuitionistic Fuzzy metric Spaces using compatible mappings of type (A)
School of Mathematics and Computer Applications, Thapar University, Patiala, India
*Corresponding author: Saurabh Manro, School of Mathematics and Computer Applications, Thapar University Patiala, India, E-mail: sauravmanro@hotmail.com
Received: December 5, 2018 Accepted: December 11, 2018 Published: December 17, 2018
Citation: Manro S. Common fixed point theorem in intuitionistic Fuzzy metric Spaces using compatible mappings of type (A). Madridge J Bioinform Syst Biol. 2018; 1(1): 5-9. doi: 10.18689/mjbsb-1000102
Copyright: © 2018 The Author(s). This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Abstract
In this paper, we prove common fixed point theorem in intuitionistic fuzzy metric space using compatible mappings of type (A).
Keywords: Intuitionistic Fuzzy metric space; Compatible mappings of type (A); Common fixed point.
AMS (2010) Subject Classification: 47H10, 54H25
Introduction
Atanassove [2] introduced and studied the concept of intuitionistic fuzzy sets as a generalization of fuzzy sets. In 2004, Park [5] defined the notion of intuitionistic fuzzy metric space with the help of continuous t-norms and continuous t-conorms. Recently, in 2006, Alaca et al.[1] using the idea of Intuitionistic fuzzy sets, defined the notion of intuitionistic fuzzy metric space with the help of continuous t-norm and continuous t- conorms as a generalization of fuzzy metric space due to Kramosil and Michalek [3]. In this paper, we prove common fixed point theorem in intuitionistic fuzzy metric space using compatible mappings of type (A).
Preliminaries
The concepts of triangular norms (t-norms) and triangular conorms (t-conorms) are known as the axiomatic skelton that we use are characterization fuzzy intersections and union respectively. These concepts were originally introduced by Menger [4] in study of statistical metric spaces.
Definition [6] A binary operation *: [0, 1]x[0, 1] → [0, 1] is continuous t-norm if * satisfies the following conditions:
(i) * is commutative and associative;
(ii) * is continuous;
(iii) a * 1 = a for all a ∈[0,1];
(iv) a * b ≤ c * d whenever a ≤ c and b ≤ d for all a, b, c, d ∈[0, 1] .
Definition [6] A binary operation ◊: [0, 1] × [0, 1] → [0, 1] is continuous t-conorm if ◊ satisfies the following conditions:
(i) ◊ is commutative and associative;
(ii) ◊ is continuous;
(iii) a ◊ 0 = a for all a ∈[0, 1];
(iv) a ◊ b ≤ c ◊ d whenever a ≤ c and b ≤ d for all a, b, c,
d ∈[0,1] .
Alaca et al. [1] using the idea of Intuitionistic fuzzy sets, defined the notion of intuitionistic fuzzy metric space with the help of continuous t-norm and continuous t-conorms as a generalization of fuzzy metric space due to Kramosil and Michalek [3] as:
Definition [1] A 5-tuple (X, M, N, *, ◊) is said to be an
intuitionistic fuzzy metric space if X is an arbitrary set, * is a
continuous t-norm, ◊ is a continuous t-conorm and M, N are
fuzzy sets on X2× [0, ∞) satisfying the following conditions:
(i) M(x, y, t) + N(x, y, t) ≤ 1 for all x, y ∈ X and t > 0;
(ii) M(x, y, 0) = 0 for all x, y ∈ X ;
(iii) M(x, y, t) = 1 for all x, y ∈ X ; and t > 0 if and only if x = y;
(iv) M(x, y, t) = M(y, x, t) for all x, y ∈ X and t > 0;
(v) M(x, y, t) * M(y, z, s) ≤ M(x, z, t + s) for all x, y, z ∈ X and
s, t > 0;
(vi) for all x, y ∈ X, M(x, y, .) : [0, ∞) → [0, 1] is left continuous;
(vii) limt→∞M(x, y, t) = 1 for all x, y ∈ X and t > 0;
(viii) N(x, y, 0) = 1 for all x, y ∈ X ;
(ix) N(x, y, t) = 0 for all x, y ∈ X and t > 0 if and only if x = y;
(x) N(x, y, t) = N(y, x, t) for all x, y ∈ X and t > 0;
(xi) N(x, y, t) ◊ N(y, z, s) ≥ N(x, z, t + s) for all x, y, z ∈ X and
s, t > 0;
(xii) for all x, y∈X, N(x, y, .) : [0, ∞)→[0, 1] is right continuous;
(xiii) limt→∞N(x, y, t) = 0 for all x, y ∈ X.
Then (M, N) is called an intuitionistic fuzzy metric space on X. The functions M(x, y, t) and N(x, y, t) denote the degree of nearness and the degree of non-nearness between x and y w.r.t. t respectively.
Remark 2.1: Every fuzzy metric space (X, M, *) is an intuitionistic fuzzy metric space of the form (X, M, 1-M, *, ◊) such that t-norm * and t-conorm ◊ are associated as x ◊ y = 1-((1-x) * (1-y)) for all x, y ∈ X.
Remark 2.2: In intuitionistic fuzzy metric space (X, M, N, *, ◊), M (x, y, *) is non-decreasing and N(x, y, ◊) is non-increasing for all x, y ∈ X.
Alaca, Turkoglu and Yildiz [1] introduced the following notions:
Definition Let (X, M, N, *, ◊) be an intuitionistic fuzzy metric
space. Then
(a) a sequence {xn} in X is said to be Cauchy sequence if, for
all t > 0 and p > 0, limn→ ∞M(xn+p, xn
, t) = 1 and limn→∞N(xn+p,
xn, t) = 0.
(b) a sequence {xn} in X is said to be convergent to a point
x∈X if, for all t > 0, limn→∞M(xn
, x, t) = 1 and limn→∞N(xn, x, t) = 0.
Definition [1] an intuitionistic fuzzy metric space (X, M, N, *, ◊) is said to be complete if and only if every Cauchy sequence in X is convergent.
Example 2.1: Let X = {1/n: n ∈ N} ∪ {0} and let * be the continuous t-norm and ◊ be the continuous t-conorm defined by a * b = ab and a ◊ b = min{1, a+b} respectively, for all a, b ∈ [0,1]. For each t ∈(0, ∞) and x, y ∈ X, define (M, N) by
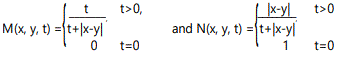
Clearly, (X, M, N, *, ◊) is complete intuitionistic fuzzy
metric space.
Definition A pair of self mappings (f, g) of a intuitionistic
fuzzy metric space (X, M, N, *, ◊) is said to be compatible if
limn→∞M(fgxn
, gfxn
, t) = 1 and limn→∞N(fgxn
, gfxn
, t) = 0 for all t
> 0, whenever {xn} is a sequence in X such that limn→∞fxn
=
limn→∞ gxn
= u for some u in X.
Definition A pair of self mappings (f, g) of a intuitionistic
fuzzy metric space (X, M, N, *,◊) is said to be compatible of
type (A) iflimn→∞M(fgxn
, ggxn
, t) = 1, limn→∞N(fgxn
, ggxn
, t) = 0
and limn→∞M(gfxn
, ffxn
, t) = 1, limn→∞N(gfxn
, ffxn
, t) = 0.
for all t > 0, whenever {xn
} is a sequence in X such that limn→∞fxn
= limn→∞ gxn
= u for some u in X.
Alaca [1] proved the following results:
Lemma Let (X, M, N, *, ◊) be intuitionistic fuzzy metric space
and for all x, y in X, t > 0 and if for a number k>1 such that
M(x, y, kt) ≤ M(x, y, t) and N(x, y, kt) ≥ N(x, y, t) Then x = y.
Lemma Let (X, M, N, *, ◊) be intuitionistic fuzzy metric space
and for all x, y in X, t > 0 and if for a number k > 1 such that

Then {yn} is a Cauchy sequence in X.
Lemma Let f and g be compatible self mappings of type (A) of a complete intuitionistic fuzzy metric space (X, M, N,*, ◊) with a *b = min{a, b} and a◊b = max{a, b} for all a, b ∈ [0,1] and fu = gu for some u ∈ X . Then gfu = fgu = ffu = ggu.
Results
Theorem: Let (X, M, N,*, ◊) be a complete intuitionistic fuzzy
metric space with a *b = min{a, b} and a◊b = max{a, b} for all
a, b ∈[0, 1]. Let A, B, S, T, P and Q be mappings from X into
itself such that the following conditions are satisfied:
(3.1) P(X) ⊆ ST (X), Q(X) ∈ AB(X),
(3.2) AB = BA, ST = TS, PB = BP, QT = TQ,
(3.3) P or AB is continuous,
(3.4) (P, AB) and (Q, ST) are pairs of compatible mappings of
type (A),
(3.5) there exist k ∈ (0,1) such that for every x, y ∈ X and t > 0
M (Px,Qy, kt) ≥ M (ABx, STy,t) * M (Px, ABx, t) * M (Qy, STy, t) *
M (Px, STy, t)
N (Px,Qy, kt) ≤ N (ABx, STy, t)◊N (Px, ABx, t) ◊N (Qy, STy, t)◊N (Px, STy, t)
Then A, B, S, T, P and Q have a unique common fixed point in X.
Proof
Forexistence:
Let x0 ∈X2
, from (3.1), there exist x1
, x2 ∈ X such that

Thus, we have
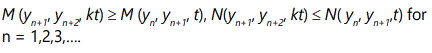
Therefore, we have
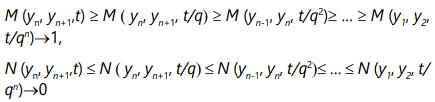
when n→∞.
For each ∈> 0 and t > 0, we can choose n0 ∈ N such that
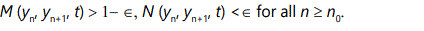
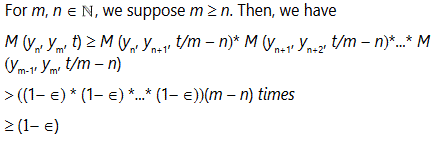
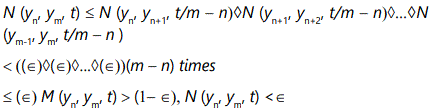
and hence {yn} is a Cauchy sequence in X. As X is complete, {yn} converges to some point
z ∈ X . Also, its subsequences converge to this point z ∈ X.

Suppose AB is continuous.
As AB is continuous, we have 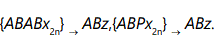
As (P, AB) is compatible pair of type (A), we have {PABx2n} → ABz.
Take x - ABx2n , y - x2n+1 in (3.5), we get
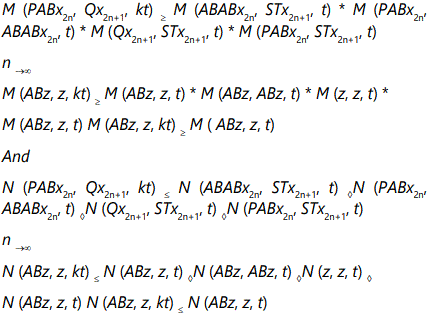
By lemma, ABz = z.
Next, we show that Pz = z.
Put x = z and y - x2n in (3.5), we get
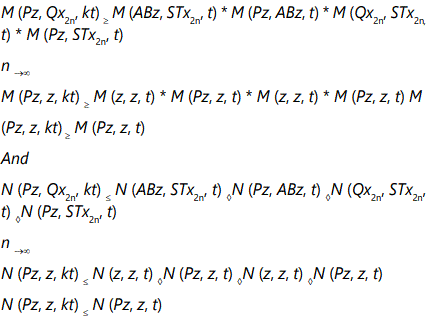
Therefore, ABz = z = Pz.
Now, we show that Bz =z.
Put x = Bz and y _ x2n-1 in (3.5), we get
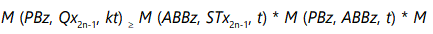
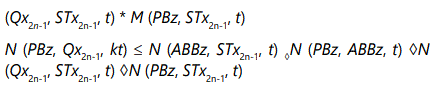
As BP = PB and AB = BA, so that
P(Bz) = (PB)z = BPz = Bz and (AB)(Bz) = (BA)(Bz) = B(AB)z = Bz.
Taking, n →∞, we get
M (Bz, z, kt) ≥ M (Bz, z, t) * M (Bz, Bz, t) * M (z, z, t) * M (Bz, z, t)
M (Bz, z, kt) ≥ M (Bz, z, t)
And
N (Bz, z, kt) ≤ N (Bz, z, t)◊N (Bz, Bz, t)◊N (z, z, t)◊N (Bz, z, t)
N (Bz, z, kt) ≤ N (Bz, z, t).
Therefore, by using lemma, we get Bz = z and also we have,
ABz = z. Therefore, Az = Bz = Pz = z.
As P(X) ⊆ ST (X), there exist u ∈ X such that z = Pz = STu.
Putting, x = x2n, y = u in (3.5), we get
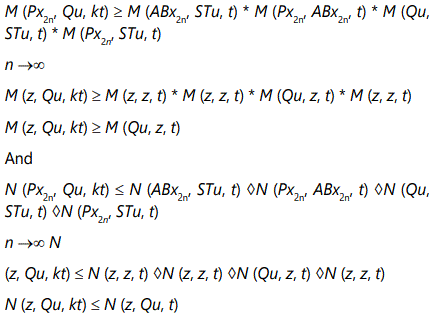
By using lemma, we get Qu = z. Hence, STu = z = Qu.
Since (Q, ST) is compatible pair of type (A), therefore, by
lemma, we have QSTu = STQu. Therefore, Qz = STz.
Now, we show that Qz = z.
Take x = x2n, y = z in (3.5), we get
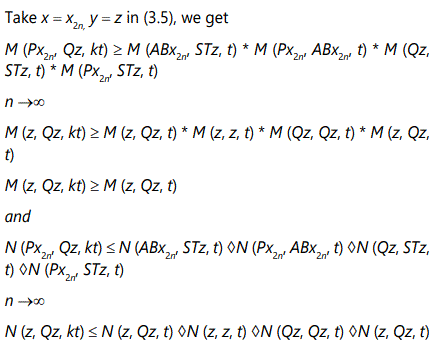
N (z, Qz, kt) = N (z, Qz, t)
Therefore, by using lemma, Qz = z.
As QT = TQ, ST = TS, we have QTz = TQz = Tz and STTz = TSTz
= TQz = Tz.
Next, we claim that Tz = z.
For this, take = x2n, y = Tz in (3.5), we get

therefore, by lemma, we get Tz = z. as STz = Qz = z = Tz. This gives, Sz =z. Hence, Az = Bz = Pz = Qz = Sz = Tz = z. Hence, z is a common fixed point of A, B, S, T, P and Q. The proof is similar P is continuous.
For uniqueness
Let u is another fixed point of A, B, S, T, P and Q. Therefore, take x = z and y = u in (3.5), we get

By lemma, we get z = u. Hence, z is a unique common fixed point of A, B, S, T, P and Q. Take B = T = I (Identity map), then theorem 3.1 becomes:
Corollary 3.1: Let (X, M, N,*, ◊) be a complete intuitionistic
fuzzy metric space with a *b = min{a, b} and a◊b = max {a, b}
for all a, b ∈ [0,1]. Let A, S, P and Q be mappings from X in to
itself such that the following conditions are satisfied:
(3.6) P(X) ⊆ S(X), Q(X) ⊆ A(X),
(3.7) P or A is continuous,
(3.8) (P, A) and (Q, S) are pairs of compatible mappings of type
(A),
(3.9) there exist k ∈(0,1) such that for every x, y ∈ X and t > 0
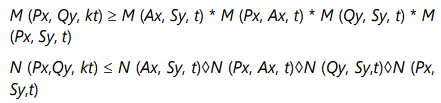
Then A, S, P and Q have a unique common fixed point in X.
References