Abstract

Preparation of graphene by chemical vapor deposition (CVD) mehtod has attracted increasing attention due to the high quality of the resulting samples. However, the relative research on diffusion and adsorption of precursor gas (the first two steps of graphene growth) on the transition metal surface is still insufficiently. In this study, three-dimensional graphene networks (3DGNs) is prepared by CVD approach with a foam nickel rod (FNR) as the template. The diffusion of CH₄ in the FNR is discussed. Then, the adsorption of CH₄ on the FNR surface is studied by the expended Langmuir equation, and the influences from H₂ on the coverage ratio of CH₄ and thickness of the resutling 3DGNs is analyzed. In order to describe the dissolution-segregation process of carbon atoms in the FNR , a parameter named “quasi-diffusivity” is proposed to avoid the tedious calculation. Based on this parameter, the relationship between the scale of 3DGNs and growth time can be simulated, and the relationship b etween the thickness of samples and their growth position can be predicted.

Keywords: Chemical Vapor Deposition; Graphene; Expanded Langmuir Equation; Quasidiffusivity.

Introduction

Since its first isolation in 2004, graphene is regarded as a star material for the dyesensitized solar cells (DSSCs) and supercapacitors fields because of its outstanding electrical and mechanical properties [1]. Recently, graphene with various morphologies including large-scale plane graphene, spherical graphene and three-dimensional graphene networks (3DGNs) have been fabricated on Cu and Ni substrates by chemical vapor deposition (CVD) method [2]. Ruoff′s group found that the deposition mechanisms of graphene on Cu and Ni surfaces are similar [3]. Besides experimental results, some theoretical mechanisms have been revealed. Zhang et al. and Meng et al. calculated the thermodynamics of graphene growth on Cu and Ni surface by first-principle calculations [4,5]. In previous reports, the attention was focused on the behavior of carbon atoms on the substrate surface. However, the diffusion and adsorption of precursor gas on the substrate surface did not arouse enough attention. In fact, these two processes are closely related to the thickness and quality of the resulting graphene. Studying on these processes is important to optimize the growth parameters and understand the growth mechanism of graphene. Recently, our group prepared high-quality 3DGNs by CVD approach with the foam nickel rod (FNR) as a template [6,7], and the 3DGNs based DSSCs and supercapacitors show high photovoltaic performance. Here, we further study the growth mechanism and kinetics of 3DGNs during the CVD process. In this study, the diffusion and adsorption properties of CH₄ in the porous FNR is discussed. The influence from H₂ on the adsorption of CH₄ and thickness of the resulting graphene is studied, as well. In order to simplify the calculation on the kinetics of graphene growth, a parameter named “quasi-diffusivity” is proposed. Based on this parameter, the relationship between scale (thickness) of the 3DGNs and growth time (growth position) can be predicted.

Material and methods

Detailed growth process of the 3DGNs has described in previous reports [6,7]. The heat preservation time are 0s, 150s, 300s, 500s, 800s and 2400s for varied samples at 1273K . An added unit was put into the reaction chamber, and the FNR were placed in the unit during the CVD process (Fig. 1, in the real case, the FNR is put into the unit).

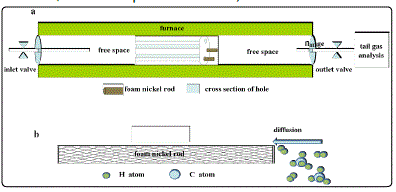
Fig. 1. (a)The schematic of the CVD system. (b) Schematic of the CH₄ and hydrogen molecules diffuse into the foam nickel rod during the heat preservation process.

Results and discussion

The scale of 3DGNs on the FNR surface increases with the extended heat preservation time (see inset of Fig. 4). No 3DGNs can be found for the sample without heat preservation step, indicating that the growth of the 3DGNs depends on the diffusion of CH₄ during the heat preservation process.

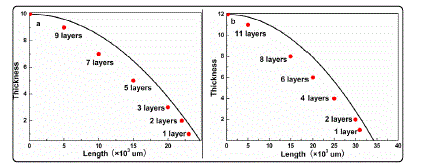
Fig. 4. (a)The schematic of the CVD system. (b) Schematic of the CH₄ and hydrogen molecules diffuse into the foam nickel rod during the heat preservation process.

Diffusion of methane molecules in the FNR
The FNR possesses a three-dimensional porous morphology with 100-150 µm in porous diameter [6,7]. The diffusion law of gas molecules in porous medium is closely related to the relationship between the mean free path of gas molecules and bore diameter of medium. According to the followed formula [8], the values of mean free path of CH₄, H₂ and Ar at 1273K are ~380, ~660 and ~470 nm, respectively.

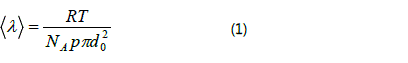
The R, T, N_A, p and d₀ are perfect gas constant, temperature, Avogadro′s constant, pressure and gas collision diameter. Due to the mean free paths of these gas molecules (atoms) are far smaller than the pore of the FNR (the ratio less than 0.01), the diffusion of them in the FNR obeys Fick′s law. For the sake of simplicity, a uniform pore structure of the FNR is assumed (~120µm in porous diameter) and the influence from its peripheral boundary is neglected. Therefore, the diffusion of CH₄ molecules in the FNR takes place along the axial direction only and the following one-dimensional partial differential equation can be employed to describe the diffuse process.

Where C, u and k are concentration, convection velocity and consumption rate of CH₄, respectively. The D^eff is the effective diffusivity of CH₄ in the FNR, which contains the pore characteristics (poriness (ε) and tortuosity (τ)) of the FNR (D^eff = Dε/τ, D represents diffusion coefficient of CH₄ in the free space). In the heat preservation process, the diffusion is an unsteady-state process and the convection velocity equals to zero. According to the results of tail gas analysis, the ∂C/∂t approximately equals a constant (a~13.6mgm^-3s^-1), thereby, the diffusion equation can be simplified into the following form in the heat preservation process:

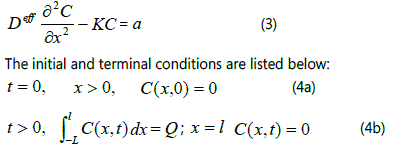
Selecting the right part of the FNR as a sample, the calculation can be performed. L is the length of free space in the right side of the unit, and l is the diffusion distance of CH₄ in the FNR. Q is the total amount of CH₄ in the free space. According to the results of tail analysis, the proportion of CH₄ in the reaction chamber is 4. 5% (the calculated value is 4.76%) before introducing the unit. After adopting the unit, the proportion of CH₄ in the free space (right part) reduces to 4.1% (the unit separates the chamber into two parts), and the proportion of CH₄ in the free space (left part) is 4.7% by calculation. A visible distinction of the CH₄ concentration appears in the right part and left part of the chamber, indicating differences in the scale of the resulting 3DGNs from the right and left parts. If the diffusion of CH₄ in these two directions is independent, the diffusivity of CH₄ in the right part of the NFR is ~10cm²s^-1 at 1273K.

Adsorption of methane molecules on the FNR surface
During the CVD process, CH₄ molecules adsorb on the surface of the FNR, which is the previous step of catalytic dehydrogenation reaction. Only the chemisorbed CH₄ can make contribution to the growth of 3DGNs, and the dehydrogenation reactions will take place for the chemisorbed CH₄ due to the catalytic effect of the substrate. According to Langmuir adsorption model, the covered fraction of adsorbent surface by adsorbate at a given temperature can be expressed as following [9]:

θ , V_∞ , b and P are coverage, maximum capacity of the adsorbent, adsorption coefficient and pressure of the adsorbate, respectively. Subscript i represents varied component, and n is the amount of components.

First of all, the adsorption amount of CH₄ molecules on the FNR surface at room temperature (300K) was detected. Six different pressures (101.325, 121.59, 141.855, 162.12, 182.385 and 202.65 KPa) were adopted, and the corresponding adsorption amounts of CH₄ are listed in the Table 1. The equation (5) can be rewritten as the following form:

Table 1 Adsorption amount of CH₄ on the FNR surface under varied pressures at 300 K.

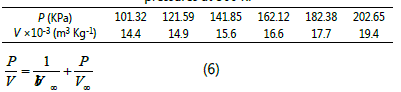
The slope and intercept are 1/V_∞ and 1/b_∞ when the P/V is plotted against the P, and the parameters b and V_∞ can be calculated according to the curve (Fig. 2). By using identical method, the parameters b and V_∞ of CH₄ under varied temperatures are calculated (Table 2).

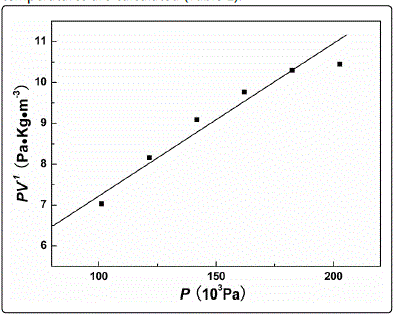
Fig. 2. PV^{-1 vs P curves of CH₄ adsorption on the FNR.}

Table 2 Adsorption parameters of CH₄ on the FNR surface under varied temperatures.

Both the b and V_∞ decrease with the increased temperature, indicating that high temperature leads to lower coverage of CH₄ on the FNR surface. The coverage values of CH₄ under varied temperatures are listed in the Table 3. In fact, the calculated values of the V_∞ and θ under high temperature exceed the actual values, and two reasons may lead to this phenomenon. Firstly, the chemical adsorbed CH₄ molecules start to decompose on the FNR surface under high temperature, then the resulted carbon atoms diffuse into the FNR and leave surface active sites to adsorb next CH₄ molecule. Moreover, nucleation reaction of CH₄ would take place in the gas phase under high temperature condition. Therefore, the V_∞ value in the Table 2 includes adsorbed and consumed CH₄ from above-mentioned two ways. However, the corresponding influence on the V_∞ is not significant because the adsorption time is faster than that of nucleation and diffusion process based on composition analysis of the tail gas.

Table 3 Coverage values of CH₄ on surface of the substrate under varied temperature.

In the absence of H₂ (the partial pressure of CH₄ and Ar are 5.7 and 95.6 KPa, respectively), the calculated coverage of CH₄ is 0.36% at 1273K. The corresponding coverage of CH₄ decreases ~10% when H₂ is added in the atmosphere (the partial pressure of argon, hydrogen and CH₄ gases are 57.4, 38.2 and 5.7 KPa, respectively), demonstrating that H₂ rather than Ar would depress the adsorption of CH₄ on the FNR surface. The adsorption of Ar atoms on the FNR is physical adsorption because Ar gas is an inert gas. Inversely, both CH₄ and H₂ molecules can be decomposed on the FNR due to the catalysis of transition metals, indicating that both the effective adsorptions of CH₄ and H₂ are chemical adsorption [10]. Xu et al. and Watwe et al. studied the adsorption heat of CH₄ and H₂ on Ni surface and calculated the needed dehydrogenation energy of them [10,11]. The similar adsorption heat and barrier height for dehydrogenation lead a competition for the surface active sites of the FNR between CH₄ and H₂. In previous reports, the influence of H₂ on graphene growth was proposed [5]: H₂ molecules reduce the roughness of Ni substrates, eliminate impurities (such as S and P) in the Ni substrates, avoid local variations in the carbon dissolvability and remove defects of graphene at high temperature. Meanwhile, thinner graphene can be prepared on Ni surface when H₂ was introduced in the atmosphere. Koskinen et al. suggested that the dangling bond of carbon atom in the graphene islands would be terminated by hydrogen atom, which limited the thickness of the resulting graphene [12].

Based on the results of this study, the reduced coverage of CH₄ shows that the influence from H₂ on graphene growth starts from the adsorption stage. The presence of H₂ not only terminates the dangling bond of graphene, but also depresses the adsorption of CH₄, which reduce the thickness of the asprepared sample.

Kinetic control step of the 3DGNs
According to the calculated diffusivity of CH₄ in the FNR (~10 cm²s^-1), CH₄ can fill the FNR in several seconds. Therefore, the 3DGNs should cover the whole surface of the FNR, which contradicts to the experimental results. There are two possible reasons can lead to this phenomenon: the CH₄ is exhausted or the dehydrogenation reaction only takes place at the endpoint of the FNR. The result demonstrates that ~0.3% CH₄ (the CH₄ concentration is 4.1% before reaction) can be found in the tail gas even the heat preservation time is as long as 2400s. Therefore, the adsorption and dehydrogenation reactions of CH₄ only happen at the endpoint part of the FNR is the fundamental reason. Diffusion velocity of CH₄ is slower than the velocity of adsorption and dehydrogenation reactions of CH₄ in the surface. The endpoint part of the FNR always possesses enough active sites to chemisorb the CH₄, and the resulting carbon atoms diffusion into the FNR both along the axial and radial directions. Thereby, the kinetic control factor of the 3DGNs growth is the diffusion of CH₄. The result indicating that the thicknesses of 3DGNs should generally reduce along the axial direction due to the concentration gradient of carbon atoms in the substrate, which is proved by our group [6,7].

Kinetic of 3DGNs growth
In the cooling process, the decreased solubility of carbon atoms in the FNR leads to the formation of graphene on substrate surface. According to the Langmuir-Mclean model [13], the surface segregation process of carbon atoms complies with the following equation:

where C_s and C_b represent the carbon atoms concentration on the surface and inner of the substrate. The ΔG_seg is the segregation free energy of carbon atom, k and T represent the Boltzmann constant and temperature, respectively. In fact, the segregation of carbon atoms is a onedimensional diffusion process and the time-dependent equation is:

where D_b is the diffusion constant. As for the initial and boundary conditions, the C_s can be assumed as zero at the beginning of the cooling process, and the initial C_b can be considered as a constant at specified depth ( C_b (z > 0,t= o) C(x) , x represent the depth). The bulk concentration of carbon atoms satisfies ₀∫¹C(x)dx = M , and the M is the total amount of carbon atoms in the FNR, which equals the change in the weight of the FNR before and after CVD process. However, the ΔG_seg is a function depending on concentration of carbon atom and temperature, which is difficult to calculate for the varied position of the FNR. Moreover, the precise concentration of carbon atoms at varied position of the FNR before and after cooling process could not to be detected. Thereby, studying the growth kinetic of the 3DGNs through precise calculation is difficult.

In order to simplify the related calculation and give a clear physical picture, we suggest a parameter named “quasidiffusivity” to describe the growth of 3DGNs. In this model, the processes of adsorption and dehydrogenation of CH₄ and the processes of diffusion and segregation of carbon atoms are deemed as an equivalent process: the 3DGNs growth in the FNR surface directly. Therefore, the relationship between the scale of 3DGNs and heat preservation time satisfies the following equation:

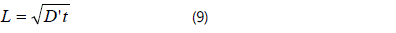
D' is the "quasi-diffusivity", which is not corresponded to any actual process.

With the increased heat preservation time, the scale of 3DGNs increases. The length of the 3DGNs enhances to 2.46 cm from 0.94 cm when the heat preservation time increases to 2400s from 300s at 1273K condition (take right part of the FNR as an example, see inset of Fig. 3a). The calculated D' is 0.0028cm²s-1 for the sample with 300s heat preservation progress, which is about one-three thousandth of CH₄ diffusivity, indicating that graphene can forms on the substrate surface only if the density of carbon atom in the FNR exceeds a certain value. By adopting the D' , the relationship between heat preservation time and scale of the resulting 3DGNs can be revealed. After comparing the calculated and practical values, it can be found that the fitting values can be used to predict the scale of the 3DGNs. However, the distinctions between the fitting and practical values become obviously when the heat preservation time is expended. Because the total amount of CH₄ is unvaried, the amount of carbon atom diffusion into the substrate in unit time gradual reduces. Therefore, the D' decreases with the increased heat preservation time. By employing the identical method, the corresponding curve of the samples in the left part is calculated ( D' ~0.0041cm²s^-1, is calculated by the sample with 300s heat preservation time, Fig. 3b). The digital image of the whole FNR after 2400s heat preservation time is displayed in the inset of Fig. 3b. The simulated and experimental results demonstrate that the length of 3DGNs can be designed by controlling heat preservation time, which is important to achieve the controllable growth of the 3DGNs. Moreover, based on the relationship between diffusivity and temperature (D~T^1.51), the scale of the 3DGNs under varied temperatures is calculated (Fig. 3).

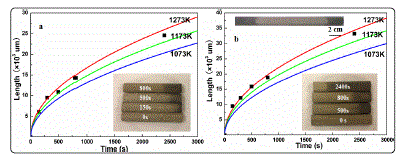
Fig. 3 Experimental and simulation results of the lengths of the 3DGNs from (a) right and (b) left sides of the FNR after varied heat preservation times.

In the cooling processes, due to carbon atoms at varied positions of the substrate possess identical segregation time, the different thicknesses of the 3DGNs results from varied densities of carbon atoms at varied positions. Based on “quasidiffusivity”, thickness of the 3DGNs at a specific position is proportional to ~ (t₀ - d²/D where t₀ and d represent heat preservation time and distance from the terminal of the FNR. Therefore, the relationship between thickness of 3DGNs and the growth position can be calculated. The simulated and real results of the 3DGNs in the two sides of the FNR with 2400s heat preservation time (1273K) are displayed in Fig. 4. The results show that the variation tendency of the thickness can be predicted by the curves, although the precision need improving. The “quasi-diffusivity” reduces with time, which results to the gradually increase deviation between the calculated and practical values. In order to obtain the better fitting results, the further optimization for the assumption and approximation is under study.

Conclusions

The diffusion and adsorption of CH₄ on the FNR is studied. The diffusion of CH₄ in the FNR complies Fick′s law. The coverage of CH₄ decrease with increased temperature, and the presence of H₂ further reduces the coverage of CH₄ due to the competition for the surface active sites. Moreover, the growth kinetic of 3DGNs is studied. The diffusion of CH₄ is found the kinetic control factor for the growth of 3DGNs. In order to simplified calculation, “quasi-diffusivity” was proposed to describe the growth process of 3DGNs. By adopting this parameter, the relationship between the scale of 3DGNs and heat preservation time can be obtained. Moreover, a preliminary study on the thickness of the prepared 3DGNs at varied position is performed. All the results manifest that the growth of 3DGNs can be predicted by “quasidiffusivity”, which is important to achieve the controllable growth of 3DGNs.

Acknowledge

This work was supported by National Natural Science Foundation of China under Grant (51506012), Jiangsu Science Foundation Fund under Grant (BK20150266); and the Basic Research Project of Changzhou City under Grant (CJ20159032).

References

1. Tang B, Hu G.X. Two kinds of graphene-based composites for photoanode applying in dye-sensitized solar cell. J Power Sources. 2012;(220): 95-102. https://dx.doi.org/10.1016/j.jpowsour.2012.07.093
2. Chen ZP, Ren CW, Gao LB, Liu BL, Pei SF, Cheng HM. Three-dimensional flexible and conductive interconnected graphene networks grown by chemical vapour deposition. Nat Mater. 2011;(10):424-428. doi: 10.1038/ nmat3001
3. Li X, Cai W, Colombo L, Ruoff RS. Evolution of graphene growth on Ni and Cu by carbon isotope labeling. Nano Lett. 2009; 9 (12):4268-4272. doi: 10.1021/nl902515k
4. Zhang WH, Wu P, Li ZY, Yang JL. First-principles thermodynamics of graphene growth on Cu surfaces. J Phys Chem C. 2011;115 (36):17782- 17787. doi: 10.1021/jp2006827.
5. Meng LJ, Sun Q, Wang JL, Ding F. Molecular dynamics simulation of chemical vapor deposition graphene growth on Ni (111) surface. J Phys Chem C. 2012;116(10):6097-6102. doi: 10.1021/jp212149c
6. Tang B, Hu GX Gaob H, Shic Z. Three-dimensional graphene network assisted high performance dye sensitized solar cells. J Power Sources. 2013; (234): 60-68. https://dx.doi.org/10.1016/j.jpowsour.2013.01.130
7. Tang B, Hu GX. Preparation of few layers three-dimensional graphene networks by CVD for energy storage application. Chem.Vapor Depos. 2014 ;20 (1-2-3):14-22. doi: 10.1002/cvde.201207052.
8. Wu SH, Wu CY. Time-resolved spatial distribution of scattered radiative energy in a two-dimensional cylindrical medium with a large mean free path for scattering. Int J Heat Mass Tran. 2001;(44):2611-2619.
9. Ignatowicz K. A mass transfer model for the adsorption of pesticide on coconut shell based activated carbon. Int. J Heat Mass Tran. 2011;54 (23- 24): 4931-4938. https://dx.doi.org/10.1016/j.ijheatmasstransfer.2011.07.005
10. Christmann K, Schober O, Ertl G., Neumann M. Adsorption of hydrogen on nickel single crystal surfaces. J Chem Phys. 1974; 60 (11): 4528-4540. doi: https://dx.doi.org/10.1063/1.1680935
11. Watwe RM, Bengaard HS, Rostrup JR, Dumesic JA, Norskov JK, Theoretical studies of stability and reactivity of CHX species on Ni (111). Journal of Catalysis. 2000;189 (1): 16-30.
12. Koskinen P, Malola S, Hakkinen H. Self-Passivating Edge Reconstructions of Graphene. Phys. Rev. Lett. 2008;101(11-12):115502-115505. doi: https://doi.org/10.1103/PhysRevLett.101.115502
13. Mccarty KF, Feibelman PJ, Loginova E, Bartelt NC. Kinetics and thermodynamics of carbon segregation and graphene growth on Ru (0001). Carbon. 2009; 47 (7)1806-1813. doi: 10.1016/j.carbon.2009.03.004